This morning we played a few games of Tic-Tac-Toe with my dd. At one point I saw her do a move that absolutely didn't benefit her. I noted to her where she should have played. She said, "But I want to let you win."
Maybe she was copying my actions (I let her win often). But this was so sweet that I let go about learning strategy and just let her let me win!
But, back to math. If you've never heard about it before, I wish to draw you attention to geometry software.
Yes, there is software for learning geometry - and it's actually a pretty neat way to learn about geometrical constructions. In fact, it can be way better than just using compass and straightedge.
Just check for yourself. Refresh your memory about constructing a circle around a given triangle. After the text there is an interactive JavaSketchPad applet illustrating the construction.
You can move the points that define the initial triangle, and see how the perpendicular bisectors move, but how they always intersect at a point that becomes the center of the circle.
To circumscribe a circle about a triangle
The site has lots more constructions with applets - just follow the links at the bottom.
So... just imagine the learning when students can build this sort of interactive construction with the software.
Tags: math, geometry
Anime, movie, comic book, video game, or TV related papercrafts, paper models and paper toys.
Friday, June 30, 2006
Geometry constructions with software plus a sweet Tic-Tac-Toe game
This morning we played a few games of Tic-Tac-Toe with my dd. At one point I saw her do a move that absolutely didn't benefit her. I noted to her where she should have played. She said, "But I want to let you win."
Maybe she was copying my actions (I let her win often). But this was so sweet that I let go about learning strategy and just let her let me win!
But, back to math. If you've never heard about it before, I wish to draw you attention to geometry software.
Yes, there is software for learning geometry - and it's actually a pretty neat way to learn about geometrical constructions. In fact, it can be way better than just using compass and straightedge.
Just check for yourself. Refresh your memory about constructing a circle around a given triangle. After the text there is an interactive JavaSketchPad applet illustrating the construction.
You can move the points that define the initial triangle, and see how the perpendicular bisectors move, but how they always intersect at a point that becomes the center of the circle.
To circumscribe a circle about a triangle
The site has lots more constructions with applets - just follow the links at the bottom.
So... just imagine the learning when students can build this sort of interactive construction with the software.
Tags: math, geometry
Maybe she was copying my actions (I let her win often). But this was so sweet that I let go about learning strategy and just let her let me win!
But, back to math. If you've never heard about it before, I wish to draw you attention to geometry software.
Yes, there is software for learning geometry - and it's actually a pretty neat way to learn about geometrical constructions. In fact, it can be way better than just using compass and straightedge.
Just check for yourself. Refresh your memory about constructing a circle around a given triangle. After the text there is an interactive JavaSketchPad applet illustrating the construction.
You can move the points that define the initial triangle, and see how the perpendicular bisectors move, but how they always intersect at a point that becomes the center of the circle.
To circumscribe a circle about a triangle
The site has lots more constructions with applets - just follow the links at the bottom.
So... just imagine the learning when students can build this sort of interactive construction with the software.
Tags: math, geometry
Wednesday, June 28, 2006
Carnival of Homeschooling, week 26
... is online at The Homeschool Cafe.
Here are a few posts that interested me: how chess helps children learn to think, tips to mastering Miquon for those who use Miquon Math, and a story of how some homeschooled students learned to write well just by reading a lot. Enjoy these and other carnival posts!
Here are a few posts that interested me: how chess helps children learn to think, tips to mastering Miquon for those who use Miquon Math, and a story of how some homeschooled students learned to write well just by reading a lot. Enjoy these and other carnival posts!
Carnival of Homeschooling, week 26
... is online at The Homeschool Cafe.
Here are a few posts that interested me: how chess helps children learn to think, tips to mastering Miquon for those who use Miquon Math, and a story of how some homeschooled students learned to write well just by reading a lot. Enjoy these and other carnival posts!
Here are a few posts that interested me: how chess helps children learn to think, tips to mastering Miquon for those who use Miquon Math, and a story of how some homeschooled students learned to write well just by reading a lot. Enjoy these and other carnival posts!
Monday, June 26, 2006
Something interesting...
A parent and licensed math teacher who started week-end homeschooling her children in math... because of poor quality of math teaching in the kids' school. She chose Singapore Math as the home replacement curriculum.
Teens and Tweens: Singapore Math
Teens and Tweens: Singapore Math
Something interesting...
A parent and licensed math teacher who started week-end homeschooling her children in math... because of poor quality of math teaching in the kids' school. She chose Singapore Math as the home replacement curriculum.
Teens and Tweens: Singapore Math
Teens and Tweens: Singapore Math
Friday, June 23, 2006
Summer contest with giveaways
I just learned today of a great online graphing site called Webgraphing.com. You can create graphs for free and analyze them too. It's the first site that I remember seeing that offers such broad range of options for analyzing the graphs.
Some functions are only available thru subscription. They offered me a free subscription, but I don't have a use for it right now, so I decided I will give it away with a blog contest!
Not only that, but I will also give away some free math ebooks of mine. So there are lots of prizes:
1) An A+ Membership to Webgraphing.com, good thru August, 2007. This is most useful for high school or beyond.
2) 3 copies of the ebook Multiplication 1 (multiplication concept and times tables)
3) 3 copies of the ebook Fractions 1 (Fraction operations such as addition, subtraction, equivalent fractions, mixed numbers)
4) 3 copies of the ebook Geometry (includes most topics of plane geometry for elementary grades).
YOU CAN ENTER the contest
1) Via email to . Please put "Blog contest" as subject line.
. Please put "Blog contest" as subject line.
2) (preferred) By linking from your blog or website. Don't forget to email me about it or leave a comment here so I will know! If you link, you will be entered thrice (three times)! Here's an example.
You can improve and improvize upon the text but it should contain all three links.
With you email, you can mention if you prefer one of the prizes in particular, because I realize it depends on the ages of your kids or students as to which prize might be the most useful.
The only thing is, I can only give away one of the subscriptions to WebGraphing.com, so if you win and you prefer that and it's already gone, then one of my ebooks will have to do.
The contest ends when 150 people have entered, or Monday July 3rd, whichever is first.
If you have questions, please comment to this post so others will see the answer too.
Some functions are only available thru subscription. They offered me a free subscription, but I don't have a use for it right now, so I decided I will give it away with a blog contest!
Not only that, but I will also give away some free math ebooks of mine. So there are lots of prizes:
1) An A+ Membership to Webgraphing.com, good thru August, 2007. This is most useful for high school or beyond.
2) 3 copies of the ebook Multiplication 1 (multiplication concept and times tables)
3) 3 copies of the ebook Fractions 1 (Fraction operations such as addition, subtraction, equivalent fractions, mixed numbers)
4) 3 copies of the ebook Geometry (includes most topics of plane geometry for elementary grades).
YOU CAN ENTER the contest
1) Via email to
 . Please put "Blog contest" as subject line.
. Please put "Blog contest" as subject line. 2) (preferred) By linking from your blog or website. Don't forget to email me about it or leave a comment here so I will know! If you link, you will be entered thrice (three times)! Here's an example.
Homeschool Math Blog is giving away an A+ Membership to Webgraphing.com and lots of math ebooks.
You can improve and improvize upon the text but it should contain all three links.
With you email, you can mention if you prefer one of the prizes in particular, because I realize it depends on the ages of your kids or students as to which prize might be the most useful.
The only thing is, I can only give away one of the subscriptions to WebGraphing.com, so if you win and you prefer that and it's already gone, then one of my ebooks will have to do.
The contest ends when 150 people have entered, or Monday July 3rd, whichever is first.
If you have questions, please comment to this post so others will see the answer too.
Summer contest with giveaways
I just learned today of a great online graphing site called Webgraphing.com. You can create graphs for free and analyze them too. It's the first site that I remember seeing that offers such broad range of options for analyzing the graphs.
Some functions are only available thru subscription. They offered me a free subscription, but I don't have a use for it right now, so I decided I will give it away with a blog contest!
Not only that, but I will also give away some free math ebooks of mine. So there are lots of prizes:
1) An A+ Membership to Webgraphing.com, good thru August, 2007. This is most useful for high school or beyond.
2) 3 copies of the ebook Multiplication 1 (multiplication concept and times tables)
3) 3 copies of the ebook Fractions 1 (Fraction operations such as addition, subtraction, equivalent fractions, mixed numbers)
4) 3 copies of the ebook Geometry (includes most topics of plane geometry for elementary grades).
YOU CAN ENTER the contest
1) Via email to . Please put "Blog contest" as subject line.
. Please put "Blog contest" as subject line.
2) (preferred) By linking from your blog or website. Don't forget to email me about it or leave a comment here so I will know! If you link, you will be entered thrice (three times)! Here's an example.
You can improve and improvize upon the text but it should contain all three links.
With you email, you can mention if you prefer one of the prizes in particular, because I realize it depends on the ages of your kids or students as to which prize might be the most useful.
The only thing is, I can only give away one of the subscriptions to WebGraphing.com, so if you win and you prefer that and it's already gone, then one of my ebooks will have to do.
The contest ends when 150 people have entered, or Monday July 3rd, whichever is first.
If you have questions, please comment to this post so others will see the answer too.
Some functions are only available thru subscription. They offered me a free subscription, but I don't have a use for it right now, so I decided I will give it away with a blog contest!
Not only that, but I will also give away some free math ebooks of mine. So there are lots of prizes:
1) An A+ Membership to Webgraphing.com, good thru August, 2007. This is most useful for high school or beyond.
2) 3 copies of the ebook Multiplication 1 (multiplication concept and times tables)
3) 3 copies of the ebook Fractions 1 (Fraction operations such as addition, subtraction, equivalent fractions, mixed numbers)
4) 3 copies of the ebook Geometry (includes most topics of plane geometry for elementary grades).
YOU CAN ENTER the contest
1) Via email to
 . Please put "Blog contest" as subject line.
. Please put "Blog contest" as subject line. 2) (preferred) By linking from your blog or website. Don't forget to email me about it or leave a comment here so I will know! If you link, you will be entered thrice (three times)! Here's an example.
Homeschool Math Blog is giving away an A+ Membership to Webgraphing.com and lots of math ebooks.
You can improve and improvize upon the text but it should contain all three links.
With you email, you can mention if you prefer one of the prizes in particular, because I realize it depends on the ages of your kids or students as to which prize might be the most useful.
The only thing is, I can only give away one of the subscriptions to WebGraphing.com, so if you win and you prefer that and it's already gone, then one of my ebooks will have to do.
The contest ends when 150 people have entered, or Monday July 3rd, whichever is first.
If you have questions, please comment to this post so others will see the answer too.
Jiji the Cat Papercraft



Here is a paper model of Jiji, the cat from Kiki's Delivery Service. This is my version of Jiji, taken with permission from Kotetsu's web site, Kinmoku.net. I used the Luna paper model (Sailormoon's cat), changed it's color and added some whiskers. Meow!
Jiji the Cat - Download
Kotetsu's Paper Models - http://www.kinmoku.net/tsukutte/
Labels:
animal,
anime,
cat,
halloween,
hayao miyazaki,
Paper Models,
Papercraft,
studio ghibli
Wednesday, June 21, 2006
Cool Blue Penguin
Summer has officially started, and it's very hot and dry here in my neck of the woods(always is). So, let's all eat some ice cream before we start on this cool blue penguin paper model. We've been featuring Mr. Ryo Tokisato's cute paper models for the past few weeks and if you like them (i know you do) kindly send him some email to thank him for his hard work.






Ryo Tokisato - tokisator [at] yahoo.co.jp
Cool Blue Penguin - Download






Ryo Tokisato - tokisator [at] yahoo.co.jp
Cool Blue Penguin - Download
Sketching sine wave
Another (student?) question along trigonometry lines:
Use a graphic calculator or an online version, such as
Function Flyer at Shodor.org.
There are more online graphing resources at my site.
You need to change the 30 degrees to radians first.
So compare 30 to 360; 30/360 is same as 1/12. So take 1/12th part of 2π, which is π/6.
Then enter the function. With the Shodor grapher, you need to use * for multiplication and x for variable, instead of t, and not use degrees but radians.
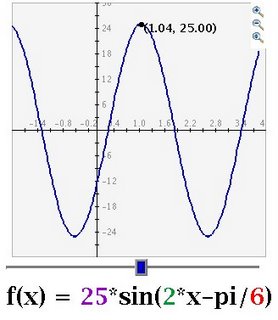
Seeing the sine graph will surely help you sketch yours on paper. Obviously the highest value is 25, the minimum value is -25.
To find its zeros, you need to think:
When does sin y = 0????
It's when y = _______ or y = _______ or when y = ______ (there are tons of these of course since it's cyclical).
Now take the argument 2t-π/6 and let it equal those numbers you found.
2t-π/6 = ___________ or 2t-π/6 = ___________ or ....
Then solve for t.
You should get π/12, 7π/12, 13π/12, 19π/12 etc. as zeros. It has zeros every
6π/12 or every π/2, and its period is π.
To find where it reaches the highest value 25 and lowest value -25, just take the exact midpoint of two consecutive zeroes. (We don't need calculus this time since it's just a simple sine).
Since midpoint of 1/12 and 7/12 is 4/12, this function has a maximum at x = 4π/12, and then a minimum at 10π/12.
P.S. I'm soon going to have a blog contest with giveaways.
Tags: math, mathematics, trigonometry
Sketch the sinusodial waveform given below over one complete cycle showing all essential values. i = 25sin(2t-30degress).
Use a graphic calculator or an online version, such as
Function Flyer at Shodor.org.
There are more online graphing resources at my site.
You need to change the 30 degrees to radians first.
So compare 30 to 360; 30/360 is same as 1/12. So take 1/12th part of 2π, which is π/6.
Then enter the function. With the Shodor grapher, you need to use * for multiplication and x for variable, instead of t, and not use degrees but radians.

Seeing the sine graph will surely help you sketch yours on paper. Obviously the highest value is 25, the minimum value is -25.
To find its zeros, you need to think:
When does sin y = 0????
It's when y = _______ or y = _______ or when y = ______ (there are tons of these of course since it's cyclical).
Now take the argument 2t-π/6 and let it equal those numbers you found.
2t-π/6 = ___________ or 2t-π/6 = ___________ or ....
Then solve for t.
You should get π/12, 7π/12, 13π/12, 19π/12 etc. as zeros. It has zeros every
6π/12 or every π/2, and its period is π.
To find where it reaches the highest value 25 and lowest value -25, just take the exact midpoint of two consecutive zeroes. (We don't need calculus this time since it's just a simple sine).
Since midpoint of 1/12 and 7/12 is 4/12, this function has a maximum at x = 4π/12, and then a minimum at 10π/12.
P.S. I'm soon going to have a blog contest with giveaways.
Tags: math, mathematics, trigonometry
Sketching sine wave
Another (student?) question along trigonometry lines:
Use a graphic calculator or an online version, such as
Function Flyer at Shodor.org.
There are more online graphing resources at my site.
You need to change the 30 degrees to radians first.
So compare 30 to 360; 30/360 is same as 1/12. So take 1/12th part of 2π, which is π/6.
Then enter the function. With the Shodor grapher, you need to use * for multiplication and x for variable, instead of t, and not use degrees but radians.

Seeing the sine graph will surely help you sketch yours on paper. Obviously the highest value is 25, the minimum value is -25.
To find its zeros, you need to think:
When does sin y = 0????
It's when y = _______ or y = _______ or when y = ______ (there are tons of these of course since it's cyclical).
Now take the argument 2t-π/6 and let it equal those numbers you found.
2t-π/6 = ___________ or 2t-π/6 = ___________ or ....
Then solve for t.
You should get π/12, 7π/12, 13π/12, 19π/12 etc. as zeros. It has zeros every
6π/12 or every π/2, and its period is π.
To find where it reaches the highest value 25 and lowest value -25, just take the exact midpoint of two consecutive zeroes. (We don't need calculus this time since it's just a simple sine).
Since midpoint of 1/12 and 7/12 is 4/12, this function has a maximum at x = 4π/12, and then a minimum at 10π/12.
P.S. I'm soon going to have a blog contest with giveaways.
Tags: math, mathematics, trigonometry
Sketch the sinusodial waveform given below over one complete cycle showing all essential values. i = 25sin(2t-30degress).
Use a graphic calculator or an online version, such as
Function Flyer at Shodor.org.
There are more online graphing resources at my site.
You need to change the 30 degrees to radians first.
So compare 30 to 360; 30/360 is same as 1/12. So take 1/12th part of 2π, which is π/6.
Then enter the function. With the Shodor grapher, you need to use * for multiplication and x for variable, instead of t, and not use degrees but radians.

Seeing the sine graph will surely help you sketch yours on paper. Obviously the highest value is 25, the minimum value is -25.
To find its zeros, you need to think:
When does sin y = 0????
It's when y = _______ or y = _______ or when y = ______ (there are tons of these of course since it's cyclical).
Now take the argument 2t-π/6 and let it equal those numbers you found.
2t-π/6 = ___________ or 2t-π/6 = ___________ or ....
Then solve for t.
You should get π/12, 7π/12, 13π/12, 19π/12 etc. as zeros. It has zeros every
6π/12 or every π/2, and its period is π.
To find where it reaches the highest value 25 and lowest value -25, just take the exact midpoint of two consecutive zeroes. (We don't need calculus this time since it's just a simple sine).
Since midpoint of 1/12 and 7/12 is 4/12, this function has a maximum at x = 4π/12, and then a minimum at 10π/12.
P.S. I'm soon going to have a blog contest with giveaways.
Tags: math, mathematics, trigonometry
Monday, June 19, 2006
Spiraling or mastery in a mathematics curriculum
Some math curricula are labeled as 'spiraling', and some are said to employ the 'mastery principle'. What does that mean, and which is better?
Spiraling mathematics curriculum introduces many topics in one grade level. It does not aim to teach those topics completely in one go, but revisits those topics the next year, the next year, and so on.
Mastery approach simply aims to teach to mastery any single topic, before going on to the next.
There is a lot of talk against spiraling math curricula, especially in regards to some reformist mathematics curricula.
So is the mastery principle then better?
Well, I think that dividing this matter into two opposite positions is a mistake. You CAN have good mathematics education employing parts of both principles.
For example, a student can learn to add 2-3 digit numbers on grade 2. She can revisit the topic on 3rd grade to learn to add 4-6 digit numbers. She can revisit the topic on 4th grade to learn to add even larger numbers.
At each grade, mastery is required - but in reality it is only partial mastery since there is more to learn about adding numbers.
Or, take fractions. You can visit the topics of fractions on 1st, 2nd, and 3rd grades just passing, noting what is a fraction, maybe having a few addition problems. Then 4th grade some more. Then on 5th grade, you study them a lot, require mastery of certain topics. On 6th, require mastery of all fraction topics. And you're done.
In essence, there is nothing wrong with spiraling. Certainly it is GOOD to present some fraction topics on lower grades, and some later on, revisit the topics next year; or study some long division on 4th and more on 5th. But we need to require mastery of these arithmetic subtopics each year. That way we can at some point easily move into ALGEBRA and leave arithmetic behind.
The approach to beware is when a mathematics topic is studied so briefly that kids don't get to master it at all. This can happen with the 'inch-deep-mile-wide' curricula that is so shock-full of topics that all a teacher can do is race thru the book...
See also:
Is your math curriculum coherent and logical?
Scope and sequence chart suggestion
Spiraling mathematics curriculum introduces many topics in one grade level. It does not aim to teach those topics completely in one go, but revisits those topics the next year, the next year, and so on.
Mastery approach simply aims to teach to mastery any single topic, before going on to the next.
There is a lot of talk against spiraling math curricula, especially in regards to some reformist mathematics curricula.
So is the mastery principle then better?
Well, I think that dividing this matter into two opposite positions is a mistake. You CAN have good mathematics education employing parts of both principles.
For example, a student can learn to add 2-3 digit numbers on grade 2. She can revisit the topic on 3rd grade to learn to add 4-6 digit numbers. She can revisit the topic on 4th grade to learn to add even larger numbers.
At each grade, mastery is required - but in reality it is only partial mastery since there is more to learn about adding numbers.
Or, take fractions. You can visit the topics of fractions on 1st, 2nd, and 3rd grades just passing, noting what is a fraction, maybe having a few addition problems. Then 4th grade some more. Then on 5th grade, you study them a lot, require mastery of certain topics. On 6th, require mastery of all fraction topics. And you're done.
In essence, there is nothing wrong with spiraling. Certainly it is GOOD to present some fraction topics on lower grades, and some later on, revisit the topics next year; or study some long division on 4th and more on 5th. But we need to require mastery of these arithmetic subtopics each year. That way we can at some point easily move into ALGEBRA and leave arithmetic behind.
Yet when it comes to the teaching of math, the divide between the two approaches may be somewhat artificial, as a spiral curriculum could be taught with a judicious eye toward mastery at every level, and any good mastery curriculum will build on concepts in a spiral fashion.
Teens and Tweens Blog, a post on Spiral Curricula (taken offline)
The approach to beware is when a mathematics topic is studied so briefly that kids don't get to master it at all. This can happen with the 'inch-deep-mile-wide' curricula that is so shock-full of topics that all a teacher can do is race thru the book...
See also:
Is your math curriculum coherent and logical?
Scope and sequence chart suggestion
Spiraling or mastery in a mathematics curriculum
Some math curricula are labeled as 'spiraling', and some are said to employ the 'mastery principle'. What does that mean, and which is better?
Spiraling mathematics curriculum introduces many topics in one grade level. It does not aim to teach those topics completely in one go, but revisits those topics the next year, the next year, and so on.
Mastery approach simply aims to teach to mastery any single topic, before going on to the next.
There is a lot of talk against spiraling math curricula, especially in regards to some reformist mathematics curricula.
So is the mastery principle then better?
Well, I think that dividing this matter into two opposite positions is a mistake. You CAN have good mathematics education employing parts of both principles.
For example, a student can learn to add 2-3 digit numbers on grade 2. She can revisit the topic on 3rd grade to learn to add 4-6 digit numbers. She can revisit the topic on 4th grade to learn to add even larger numbers.
At each grade, mastery is required - but in reality it is only partial mastery since there is more to learn about adding numbers.
Or, take fractions. You can visit the topics of fractions on 1st, 2nd, and 3rd grades just passing, noting what is a fraction, maybe having a few addition problems. Then 4th grade some more. Then on 5th grade, you study them a lot, require mastery of certain topics. On 6th, require mastery of all fraction topics. And you're done.
In essence, there is nothing wrong with spiraling. Certainly it is GOOD to present some fraction topics on lower grades, and some later on, revisit the topics next year; or study some long division on 4th and more on 5th. But we need to require mastery of these arithmetic subtopics each year. That way we can at some point easily move into ALGEBRA and leave arithmetic behind.
The approach to beware is when a mathematics topic is studied so briefly that kids don't get to master it at all. This can happen with the 'inch-deep-mile-wide' curricula that is so shock-full of topics that all a teacher can do is race thru the book...
See also:
Is your math curriculum coherent and logical?
Scope and sequence chart suggestion
Spiraling mathematics curriculum introduces many topics in one grade level. It does not aim to teach those topics completely in one go, but revisits those topics the next year, the next year, and so on.
Mastery approach simply aims to teach to mastery any single topic, before going on to the next.
There is a lot of talk against spiraling math curricula, especially in regards to some reformist mathematics curricula.
So is the mastery principle then better?
Well, I think that dividing this matter into two opposite positions is a mistake. You CAN have good mathematics education employing parts of both principles.
For example, a student can learn to add 2-3 digit numbers on grade 2. She can revisit the topic on 3rd grade to learn to add 4-6 digit numbers. She can revisit the topic on 4th grade to learn to add even larger numbers.
At each grade, mastery is required - but in reality it is only partial mastery since there is more to learn about adding numbers.
Or, take fractions. You can visit the topics of fractions on 1st, 2nd, and 3rd grades just passing, noting what is a fraction, maybe having a few addition problems. Then 4th grade some more. Then on 5th grade, you study them a lot, require mastery of certain topics. On 6th, require mastery of all fraction topics. And you're done.
In essence, there is nothing wrong with spiraling. Certainly it is GOOD to present some fraction topics on lower grades, and some later on, revisit the topics next year; or study some long division on 4th and more on 5th. But we need to require mastery of these arithmetic subtopics each year. That way we can at some point easily move into ALGEBRA and leave arithmetic behind.
Yet when it comes to the teaching of math, the divide between the two approaches may be somewhat artificial, as a spiral curriculum could be taught with a judicious eye toward mastery at every level, and any good mastery curriculum will build on concepts in a spiral fashion.
Teens and Tweens Blog, a post on Spiral Curricula (taken offline)
The approach to beware is when a mathematics topic is studied so briefly that kids don't get to master it at all. This can happen with the 'inch-deep-mile-wide' curricula that is so shock-full of topics that all a teacher can do is race thru the book...
See also:
Is your math curriculum coherent and logical?
Scope and sequence chart suggestion
Friday, June 16, 2006
Enjoying geometry proofs
Here recently I finished reading the two Dr. Math geometry books. (I will be writing a review of them, and I can say they're pretty good & inexpensive books!)
The one meant for high school geometry had as its last chapter circles and theorems about circles. Well circles never were the strongest part of my mathematical knowledge, for whatever reason... (I think it stems from the fact that so big part of school geometry concentrates on calculating areas and volumes, and not on properties of figures.)
So I wanted to brush up on circle theorems. I quickly read over the circle chapter on another geometry book I have, called "Geometry: A Guided Inquiry". (I can recommend that book as well. It often asks the student to explore and try find theorems. See more info here.)
I don't know about you, but to me, reading & learning theorems and proofs can be enjoyable. First you maybe struggle to grasp it all, but afterwards there is a great satisfying feeling and admiration of the logical process - and it's BEAUTIFUL.
So I thought I'd blog about inscribed angles... so you see a tiny glimpse of this logical process of proof.
1) Let's suppose we've already proved this theorem: that inscribed angles are equal, and are half of the corresponding central angle.

All these angles A are equal (or congruent as they say), and are half of angle B.
I can even draw many of them in the same pic: now the red, blue, black, and green angles have the same measure, exactly half of angle B.

(As a sideline, if we make B to be 180 degrees so it forms a diameter, then what is the measure of A?)
For proof of this theorem, see Inscribed and Central Angles in a Circle: What is this about? A Mathematical Droodle - a nice interactive applet shows the angle relationship, too.
2) THEN, after understanding that, let's consider two chords in a circle that intersect.

Filling in some more lines, we get what I'd call the "slanted timeglass figure".
We see there are two inscribed angles here, from the same points, and they're congruent (angles A and A').
Angles B and B' are congruent since they're vertical angles.
SO.... even the third angles of these two triangles must be equal (since angles in a triangle always add up to 180 degrees).
It follows that we have two SIMILAR TRIANGLES! We have just PROVEN that the time glass figure inside a circle consists of two similar (same shape) triangles.
Well that was just a tiny tiny glimpse, but I hope you see how this process could work. And I wish our students can get these tiny glimpses even BEFORE high school geometry - to learn appreciate math a little more, to see its beauty in action, to learn what proving is all about. In my mind, proofs are NOT something boring - they're part of the beauty of mathematics.
Tags: math, geometry
The one meant for high school geometry had as its last chapter circles and theorems about circles. Well circles never were the strongest part of my mathematical knowledge, for whatever reason... (I think it stems from the fact that so big part of school geometry concentrates on calculating areas and volumes, and not on properties of figures.)
So I wanted to brush up on circle theorems. I quickly read over the circle chapter on another geometry book I have, called "Geometry: A Guided Inquiry". (I can recommend that book as well. It often asks the student to explore and try find theorems. See more info here.)
I don't know about you, but to me, reading & learning theorems and proofs can be enjoyable. First you maybe struggle to grasp it all, but afterwards there is a great satisfying feeling and admiration of the logical process - and it's BEAUTIFUL.
So I thought I'd blog about inscribed angles... so you see a tiny glimpse of this logical process of proof.
1) Let's suppose we've already proved this theorem: that inscribed angles are equal, and are half of the corresponding central angle.

All these angles A are equal (or congruent as they say), and are half of angle B.
I can even draw many of them in the same pic: now the red, blue, black, and green angles have the same measure, exactly half of angle B.

(As a sideline, if we make B to be 180 degrees so it forms a diameter, then what is the measure of A?)
For proof of this theorem, see Inscribed and Central Angles in a Circle: What is this about? A Mathematical Droodle - a nice interactive applet shows the angle relationship, too.
2) THEN, after understanding that, let's consider two chords in a circle that intersect.

Filling in some more lines, we get what I'd call the "slanted timeglass figure".
We see there are two inscribed angles here, from the same points, and they're congruent (angles A and A').
Angles B and B' are congruent since they're vertical angles.
SO.... even the third angles of these two triangles must be equal (since angles in a triangle always add up to 180 degrees).
It follows that we have two SIMILAR TRIANGLES! We have just PROVEN that the time glass figure inside a circle consists of two similar (same shape) triangles.
Well that was just a tiny tiny glimpse, but I hope you see how this process could work. And I wish our students can get these tiny glimpses even BEFORE high school geometry - to learn appreciate math a little more, to see its beauty in action, to learn what proving is all about. In my mind, proofs are NOT something boring - they're part of the beauty of mathematics.
Tags: math, geometry
Enjoying geometry proofs
Here recently I finished reading the two Dr. Math geometry books. (I will be writing a review of them, and I can say they're pretty good & inexpensive books!)
The one meant for high school geometry had as its last chapter circles and theorems about circles. Well circles never were the strongest part of my mathematical knowledge, for whatever reason... (I think it stems from the fact that so big part of school geometry concentrates on calculating areas and volumes, and not on properties of figures.)
So I wanted to brush up on circle theorems. I quickly read over the circle chapter on another geometry book I have, called "Geometry: A Guided Inquiry". (I can recommend that book as well. It often asks the student to explore and try find theorems. See more info here.)
I don't know about you, but to me, reading & learning theorems and proofs can be enjoyable. First you maybe struggle to grasp it all, but afterwards there is a great satisfying feeling and admiration of the logical process - and it's BEAUTIFUL.
So I thought I'd blog about inscribed angles... so you see a tiny glimpse of this logical process of proof.
1) Let's suppose we've already proved this theorem: that inscribed angles are equal, and are half of the corresponding central angle.

All these angles A are equal (or congruent as they say), and are half of angle B.
I can even draw many of them in the same pic: now the red, blue, black, and green angles have the same measure, exactly half of angle B.

(As a sideline, if we make B to be 180 degrees so it forms a diameter, then what is the measure of A?)
For proof of this theorem, see Inscribed and Central Angles in a Circle: What is this about? A Mathematical Droodle - a nice interactive applet shows the angle relationship, too.
2) THEN, after understanding that, let's consider two chords in a circle that intersect.

Filling in some more lines, we get what I'd call the "slanted timeglass figure".
We see there are two inscribed angles here, from the same points, and they're congruent (angles A and A').
Angles B and B' are congruent since they're vertical angles.
SO.... even the third angles of these two triangles must be equal (since angles in a triangle always add up to 180 degrees).
It follows that we have two SIMILAR TRIANGLES! We have just PROVEN that the time glass figure inside a circle consists of two similar (same shape) triangles.
Well that was just a tiny tiny glimpse, but I hope you see how this process could work. And I wish our students can get these tiny glimpses even BEFORE high school geometry - to learn appreciate math a little more, to see its beauty in action, to learn what proving is all about. In my mind, proofs are NOT something boring - they're part of the beauty of mathematics.
Tags: math, geometry
The one meant for high school geometry had as its last chapter circles and theorems about circles. Well circles never were the strongest part of my mathematical knowledge, for whatever reason... (I think it stems from the fact that so big part of school geometry concentrates on calculating areas and volumes, and not on properties of figures.)
So I wanted to brush up on circle theorems. I quickly read over the circle chapter on another geometry book I have, called "Geometry: A Guided Inquiry". (I can recommend that book as well. It often asks the student to explore and try find theorems. See more info here.)
I don't know about you, but to me, reading & learning theorems and proofs can be enjoyable. First you maybe struggle to grasp it all, but afterwards there is a great satisfying feeling and admiration of the logical process - and it's BEAUTIFUL.
So I thought I'd blog about inscribed angles... so you see a tiny glimpse of this logical process of proof.
1) Let's suppose we've already proved this theorem: that inscribed angles are equal, and are half of the corresponding central angle.

All these angles A are equal (or congruent as they say), and are half of angle B.
I can even draw many of them in the same pic: now the red, blue, black, and green angles have the same measure, exactly half of angle B.

(As a sideline, if we make B to be 180 degrees so it forms a diameter, then what is the measure of A?)
For proof of this theorem, see Inscribed and Central Angles in a Circle: What is this about? A Mathematical Droodle - a nice interactive applet shows the angle relationship, too.
2) THEN, after understanding that, let's consider two chords in a circle that intersect.

Filling in some more lines, we get what I'd call the "slanted timeglass figure".
We see there are two inscribed angles here, from the same points, and they're congruent (angles A and A').
Angles B and B' are congruent since they're vertical angles.
SO.... even the third angles of these two triangles must be equal (since angles in a triangle always add up to 180 degrees).
It follows that we have two SIMILAR TRIANGLES! We have just PROVEN that the time glass figure inside a circle consists of two similar (same shape) triangles.
Well that was just a tiny tiny glimpse, but I hope you see how this process could work. And I wish our students can get these tiny glimpses even BEFORE high school geometry - to learn appreciate math a little more, to see its beauty in action, to learn what proving is all about. In my mind, proofs are NOT something boring - they're part of the beauty of mathematics.
Tags: math, geometry
Thursday, June 15, 2006
No-Face 2
Here's the update to the No-Face paper model, it's about 2x bigger and I've cleaned up the lines on its face for a more cleaner look. What do you guys think? what else should be changed or added?






NoFace - Download






NoFace - Download
Labels:
anime,
hayao miyazaki,
Paper Models,
Papercraft,
studio ghibli
Wednesday, June 14, 2006
No-Face
It's been pretty quite in paperkraft land for the past couple weeks, not because I've run out of paper models to do, but I've been doing my very own paper model. I won't go into details that much but let me tell you about the software I used, I bought Newtek Lightwave 8.5 as my 3D modeler and Tamasoft's Pepakura Designer to convert the 3D object to a paper model. I've chosen No-Face (Spirited Away) as my first model because it was the most requested via email and also I think it's pretty easy???
As you can see from the pics below that the final model is a bit small and still has that blocky effect all over his face and body, I'm still in the process of refining those features and making him about 2 or 3 times bigger. Also, I have not finished the instructions for assembling him (for novices), but most of you shouldn't have any problems with this one, I've pretty much aligned up the parts to be connected. This paper model is about 3 1/2" x 1 1/2" when completed.
So, I hope you like it and keep updated for the "smoother" version coming soon.





NoFace - Download
As you can see from the pics below that the final model is a bit small and still has that blocky effect all over his face and body, I'm still in the process of refining those features and making him about 2 or 3 times bigger. Also, I have not finished the instructions for assembling him (for novices), but most of you shouldn't have any problems with this one, I've pretty much aligned up the parts to be connected. This paper model is about 3 1/2" x 1 1/2" when completed.
So, I hope you like it and keep updated for the "smoother" version coming soon.






NoFace - Download
Labels:
anime,
hayao miyazaki,
Paper Models,
Papercraft,
studio ghibli
Adding 2-digit numbers - followup
So today I experimented, and gave my daughter a problem 15 + 25 for starters, to see what she'd do.
1 5
+ 2 5

3 10
She promptly wrote down 3 and 10. Then she looked at the numbers, kind of wondering, saying "It's ten three." I asked her how would we say the number, pointing with my finger and making a sweeping motion from 3 towards 10, and then she said, "Thirty-ten."
I said yes, it's thirty-ten, but we say it differently normally. Then I asked her to find it out using abacus. She easily found it was 40. Then we erased her numbers and wrote in 40.
She did two more similar problems on her own, first adding tens, then ones (as her custom seems to be), and then erasing the numbers and changing them to the right result. She seemed very happy for being able to understand the gimmick.
So we did all that without discussing 'carrying' or putting little number 1 up on top, or anything.
I figure next time I'll try a problem where the ones add up to 11 or 12.
1 5
+ 2 5
3 10
She promptly wrote down 3 and 10. Then she looked at the numbers, kind of wondering, saying "It's ten three." I asked her how would we say the number, pointing with my finger and making a sweeping motion from 3 towards 10, and then she said, "Thirty-ten."
I said yes, it's thirty-ten, but we say it differently normally. Then I asked her to find it out using abacus. She easily found it was 40. Then we erased her numbers and wrote in 40.
She did two more similar problems on her own, first adding tens, then ones (as her custom seems to be), and then erasing the numbers and changing them to the right result. She seemed very happy for being able to understand the gimmick.
So we did all that without discussing 'carrying' or putting little number 1 up on top, or anything.
I figure next time I'll try a problem where the ones add up to 11 or 12.
Adding 2-digit numbers - followup
So today I experimented, and gave my daughter a problem 15 + 25 for starters, to see what she'd do.
1 5
+ 2 5

3 10
She promptly wrote down 3 and 10. Then she looked at the numbers, kind of wondering, saying "It's ten three." I asked her how would we say the number, pointing with my finger and making a sweeping motion from 3 towards 10, and then she said, "Thirty-ten."
I said yes, it's thirty-ten, but we say it differently normally. Then I asked her to find it out using abacus. She easily found it was 40. Then we erased her numbers and wrote in 40.
She did two more similar problems on her own, first adding tens, then ones (as her custom seems to be), and then erasing the numbers and changing them to the right result. She seemed very happy for being able to understand the gimmick.
So we did all that without discussing 'carrying' or putting little number 1 up on top, or anything.
I figure next time I'll try a problem where the ones add up to 11 or 12.
1 5
+ 2 5
3 10
She promptly wrote down 3 and 10. Then she looked at the numbers, kind of wondering, saying "It's ten three." I asked her how would we say the number, pointing with my finger and making a sweeping motion from 3 towards 10, and then she said, "Thirty-ten."
I said yes, it's thirty-ten, but we say it differently normally. Then I asked her to find it out using abacus. She easily found it was 40. Then we erased her numbers and wrote in 40.
She did two more similar problems on her own, first adding tens, then ones (as her custom seems to be), and then erasing the numbers and changing them to the right result. She seemed very happy for being able to understand the gimmick.
So we did all that without discussing 'carrying' or putting little number 1 up on top, or anything.
I figure next time I'll try a problem where the ones add up to 11 or 12.
Tuesday, June 13, 2006
Adding 2-digit numbers
Just today I made my dd some more math problems with adding 2-digit numbers - the easy type where you don't have to carry. She's getting it now pretty good.
I always talk about adding so many tens to so many tens so she won't forget it's about adding tens and adding ones. Somehow she's doing the tens first, then the ones, and she tends to say, after adding tens:
52
+ 37

8
"Oh, it's going to be eighty-something."
You know, that's the way many people add numbers mentally: 52 + 37, we add tens first and get 80. Then add ones.
Even with 46 + 36, you probably add tens first and get 70, then add ones and go over to 82.
So a thought hit my mind: one day I will present to her a problem where the ones make a ten, such as
26
+ 34

Is she going to get an answer fifty-ten?
Well, that's what it is, basically. We just need to recognize that's not the normal way of saying it but that we need to add that ten to the fifty so it becomes sixty.
Anyway, I really feel I'm going to experiment with something like that soon. And I wonder if doing it this way would make it more obvious why we need to 'carry'.
You see, if you first add the ones, you don't really yet know what you're coming to and so the whole addition is more 'uncertain'.
26
+ 58

14
Maybe the child adds ones and gets 14, and feels perfectly happy about that.
But if you add tens first and are expecting a single-digit number, would it make it easier to grasp that the tens number needs to change??
Or, here's one more 'variation':
26
+ 58

14
Then add tens... get seventy... and erase the one of the 14 and replace it with 8. This, of course, would just be an alternate way of 'carrying'.
26
+ 58

84
What do you think? Or maybe it's a matter of, well it won't matter too much whichever way, as long as the end result is the same and we learn our math.
Tags: math, elementary
I always talk about adding so many tens to so many tens so she won't forget it's about adding tens and adding ones. Somehow she's doing the tens first, then the ones, and she tends to say, after adding tens:
52
+ 37
8
"Oh, it's going to be eighty-something."
You know, that's the way many people add numbers mentally: 52 + 37, we add tens first and get 80. Then add ones.
Even with 46 + 36, you probably add tens first and get 70, then add ones and go over to 82.
So a thought hit my mind: one day I will present to her a problem where the ones make a ten, such as
26
+ 34
Is she going to get an answer fifty-ten?
Well, that's what it is, basically. We just need to recognize that's not the normal way of saying it but that we need to add that ten to the fifty so it becomes sixty.
Anyway, I really feel I'm going to experiment with something like that soon. And I wonder if doing it this way would make it more obvious why we need to 'carry'.
You see, if you first add the ones, you don't really yet know what you're coming to and so the whole addition is more 'uncertain'.
26
+ 58
14
Maybe the child adds ones and gets 14, and feels perfectly happy about that.
But if you add tens first and are expecting a single-digit number, would it make it easier to grasp that the tens number needs to change??
Or, here's one more 'variation':
26
+ 58
14
Then add tens... get seventy... and erase the one of the 14 and replace it with 8. This, of course, would just be an alternate way of 'carrying'.
26
+ 58
84
What do you think? Or maybe it's a matter of, well it won't matter too much whichever way, as long as the end result is the same and we learn our math.
Tags: math, elementary
Adding 2-digit numbers
Just today I made my dd some more math problems with adding 2-digit numbers - the easy type where you don't have to carry. She's getting it now pretty good.
I always talk about adding so many tens to so many tens so she won't forget it's about adding tens and adding ones. Somehow she's doing the tens first, then the ones, and she tends to say, after adding tens:
52
+ 37

8
"Oh, it's going to be eighty-something."
You know, that's the way many people add numbers mentally: 52 + 37, we add tens first and get 80. Then add ones.
Even with 46 + 36, you probably add tens first and get 70, then add ones and go over to 82.
So a thought hit my mind: one day I will present to her a problem where the ones make a ten, such as
26
+ 34

Is she going to get an answer fifty-ten?
Well, that's what it is, basically. We just need to recognize that's not the normal way of saying it but that we need to add that ten to the fifty so it becomes sixty.
Anyway, I really feel I'm going to experiment with something like that soon. And I wonder if doing it this way would make it more obvious why we need to 'carry'.
You see, if you first add the ones, you don't really yet know what you're coming to and so the whole addition is more 'uncertain'.
26
+ 58

14
Maybe the child adds ones and gets 14, and feels perfectly happy about that.
But if you add tens first and are expecting a single-digit number, would it make it easier to grasp that the tens number needs to change??
Or, here's one more 'variation':
26
+ 58

14
Then add tens... get seventy... and erase the one of the 14 and replace it with 8. This, of course, would just be an alternate way of 'carrying'.
26
+ 58

84
What do you think? Or maybe it's a matter of, well it won't matter too much whichever way, as long as the end result is the same and we learn our math.
Tags: math, elementary
I always talk about adding so many tens to so many tens so she won't forget it's about adding tens and adding ones. Somehow she's doing the tens first, then the ones, and she tends to say, after adding tens:
52
+ 37
8
"Oh, it's going to be eighty-something."
You know, that's the way many people add numbers mentally: 52 + 37, we add tens first and get 80. Then add ones.
Even with 46 + 36, you probably add tens first and get 70, then add ones and go over to 82.
So a thought hit my mind: one day I will present to her a problem where the ones make a ten, such as
26
+ 34
Is she going to get an answer fifty-ten?
Well, that's what it is, basically. We just need to recognize that's not the normal way of saying it but that we need to add that ten to the fifty so it becomes sixty.
Anyway, I really feel I'm going to experiment with something like that soon. And I wonder if doing it this way would make it more obvious why we need to 'carry'.
You see, if you first add the ones, you don't really yet know what you're coming to and so the whole addition is more 'uncertain'.
26
+ 58
14
Maybe the child adds ones and gets 14, and feels perfectly happy about that.
But if you add tens first and are expecting a single-digit number, would it make it easier to grasp that the tens number needs to change??
Or, here's one more 'variation':
26
+ 58
14
Then add tens... get seventy... and erase the one of the 14 and replace it with 8. This, of course, would just be an alternate way of 'carrying'.
26
+ 58
84
What do you think? Or maybe it's a matter of, well it won't matter too much whichever way, as long as the end result is the same and we learn our math.
Tags: math, elementary
Sunday, June 11, 2006
Saturday, June 10, 2006
Measurement projects
I have a feeling blogging might slow down over the summer...
I've been dealing with math daily, though (of course). I'm writing a collection of worksheets for a company, and teaching too.
The last few days have been spent with writing an answer key. I thought I'd post a few problems here for your enjoyment - sort of measuring projects. Maybe you can involve your kids in some hands-on splish splosh splash water experiments and learn geometry too. :)
Tags: math, elementary, geometry
I've been dealing with math daily, though (of course). I'm writing a collection of worksheets for a company, and teaching too.
The last few days have been spent with writing an answer key. I thought I'd post a few problems here for your enjoyment - sort of measuring projects. Maybe you can involve your kids in some hands-on splish splosh splash water experiments and learn geometry too. :)
1. You need: an empty cylinder (for example from inside a toilet paper roll), a small ball, and a tennis ball.
The small ball should fit inside the cylinder fairly snugly, and the tennis ball shouldn't.
a. What should you measure from the ball and the cylinder to know whether a ball fits, without actually trying it out?
b. Find how many balls you could stack inside the cylinder, if you had more. Think what measurements and calculations you should do to find that out.
c. How many of the small balls would fit inside a cylinder that is 1 m 34 cm tall?
d. How many tennis balls would fit inside a cylinder that is 1 m 34 cm tall?
2. You need: a measuring cup that measures in milliliters, water, a small ball, a tennis ball, a drinking glass.
Figure out a method to find out the volume of the balls. After all, you cannot pour water inside them.
The volumes are:
the small ball _______ml,
the tennis ball _______ml,
the drinking glass _______ml.
The volume of the small ball is about ____________of the volume of the drinking glass.
The volume of the tennis ball is about ____________of the volume of the drinking glass.
3. How could you find out the volume of the toilet paper roll cylinder? Is it more than a glass of water?
4. You need: some baby blocks, some dice, a small cardboard box.
Experiment, measure, and find a method so you can find the answers.
a. How many baby blocks would fit inside the box?
b. How many dice would fit inside the box?
Tags: math, elementary, geometry
Measurement projects
I have a feeling blogging might slow down over the summer...
I've been dealing with math daily, though (of course). I'm writing a collection of worksheets for a company, and teaching too.
The last few days have been spent with writing an answer key. I thought I'd post a few problems here for your enjoyment - sort of measuring projects. Maybe you can involve your kids in some hands-on splish splosh splash water experiments and learn geometry too. :)
Tags: math, elementary, geometry
I've been dealing with math daily, though (of course). I'm writing a collection of worksheets for a company, and teaching too.
The last few days have been spent with writing an answer key. I thought I'd post a few problems here for your enjoyment - sort of measuring projects. Maybe you can involve your kids in some hands-on splish splosh splash water experiments and learn geometry too. :)
1. You need: an empty cylinder (for example from inside a toilet paper roll), a small ball, and a tennis ball.
The small ball should fit inside the cylinder fairly snugly, and the tennis ball shouldn't.
a. What should you measure from the ball and the cylinder to know whether a ball fits, without actually trying it out?
b. Find how many balls you could stack inside the cylinder, if you had more. Think what measurements and calculations you should do to find that out.
c. How many of the small balls would fit inside a cylinder that is 1 m 34 cm tall?
d. How many tennis balls would fit inside a cylinder that is 1 m 34 cm tall?
2. You need: a measuring cup that measures in milliliters, water, a small ball, a tennis ball, a drinking glass.
Figure out a method to find out the volume of the balls. After all, you cannot pour water inside them.
The volumes are:
the small ball _______ml,
the tennis ball _______ml,
the drinking glass _______ml.
The volume of the small ball is about ____________of the volume of the drinking glass.
The volume of the tennis ball is about ____________of the volume of the drinking glass.
3. How could you find out the volume of the toilet paper roll cylinder? Is it more than a glass of water?
4. You need: some baby blocks, some dice, a small cardboard box.
Experiment, measure, and find a method so you can find the answers.
a. How many baby blocks would fit inside the box?
b. How many dice would fit inside the box?
Tags: math, elementary, geometry
Wednesday, June 7, 2006
Links
Paperkraft Sites - links to the papercrafters featured on this site.
3 Eyed Bear
Bamboogila's Corner
Bryan Rollins
Burlabox
Canon Creative Park
CeraCera Papercraft Automata
Cubeecraft
Custom Paper Toys
Desktop Gremlins
Dolly Oblong
Epson Papercraft Mania!
Flying Pig
Harlancore
Haunted Dimensions
Horrorwood
Ichinogami Papercraft
Icthus7 Papercraft
Imprime Recorta y Pega
Jonny Chiba
Kawaii-Style
Little Paper Life
Kaizo Papercrafts
Kamimodel
Kei Craft Studio
Litro-Craft
Loulou & Tummie
Mabikaze Paperworks Northarant
Makula.tv
Mascal.it
Marshall Alexander
Matthijs Kamstra
Morgan Gleave Art & Design
Nanibird
Nicebunny
Nice Paper Toys
Ninjatoes
Nintendo Papercraft
Oh-Sheet
Paper and Glue
Paperboxworld
Paper Inside
Paper Invaders
Paperpino
Paper Pokes
Paper Poseables
Paper Toy Bonanza
Papertoys.info
Paper Replika
Pepakura.net
Pixtoyz
Planet StarFreak
Professor Plastik
Ryo Tokisato
Sal Azad
SD-Sascha Seite
Speakerdog Paper Toys
Shin Tanaka
The Papercraft Couple
Yamaha Motors Papercraft
Paperkraft Friends - if my site doesn't have the link to a papercraft you're looking for, then these sites surely have them.
Il Favoloso Mondo Di Carta Di Toto
Cubeecraft Fan Club
Goblin Paper
FX Console
Papercraft Museum
Navi's Paperkraft
Paper Forest
Papercraft Paradise
3 Eyed Bear
Bamboogila's Corner
Bryan Rollins
Burlabox
Canon Creative Park
CeraCera Papercraft Automata
Cubeecraft
Custom Paper Toys
Desktop Gremlins
Dolly Oblong
Epson Papercraft Mania!
Flying Pig
Harlancore
Haunted Dimensions
Horrorwood
Ichinogami Papercraft
Icthus7 Papercraft
Imprime Recorta y Pega
Jonny Chiba
Kawaii-Style
Little Paper Life
Kaizo Papercrafts
Kamimodel
Kei Craft Studio
Litro-Craft
Loulou & Tummie
Mabikaze Paperworks Northarant
Makula.tv
Mascal.it
Marshall Alexander
Matthijs Kamstra
Morgan Gleave Art & Design
Nanibird
Nicebunny
Nice Paper Toys
Ninjatoes
Nintendo Papercraft
Oh-Sheet
Paper and Glue
Paperboxworld
Paper Inside
Paper Invaders
Paperpino
Paper Pokes
Paper Poseables
Paper Toy Bonanza
Papertoys.info
Paper Replika
Pepakura.net
Pixtoyz
Planet StarFreak
Professor Plastik
Ryo Tokisato
Sal Azad
SD-Sascha Seite
Speakerdog Paper Toys
Shin Tanaka
The Papercraft Couple
Yamaha Motors Papercraft
Paperkraft Friends - if my site doesn't have the link to a papercraft you're looking for, then these sites surely have them.
Il Favoloso Mondo Di Carta Di Toto
Cubeecraft Fan Club
Goblin Paper
FX Console
Papercraft Museum
Navi's Paperkraft
Paper Forest
Papercraft Paradise
Tuesday, June 6, 2006
Homeschooling Carnival
This week the Carnival of Homescooling is held at palmtreepundit.blogspot.com. There were several math-related posts (including mine), again! I so delighted in reading and realizing that homeschoolers are appreciating mathematics and treating it 'positively'.
This is so important, as I've stressed many times. Our attitudes towards math (or history!) influence our students. An enthusiastic teacher is a big part of learning to enjoy a subject.
I am sincerely looking forward to re-learning geography, for example, with my kids. Here's a pick from the carnival: seems like US youngsters do not know geography very well... I think it is a fascinating subject, to learn about all the variety in the ways people live on this globe, and the variety of landscapes and land formations, or how volcanos or earthquakes work. I hope you can tell that I enjoy learning!
This is so important, as I've stressed many times. Our attitudes towards math (or history!) influence our students. An enthusiastic teacher is a big part of learning to enjoy a subject.
I am sincerely looking forward to re-learning geography, for example, with my kids. Here's a pick from the carnival: seems like US youngsters do not know geography very well... I think it is a fascinating subject, to learn about all the variety in the ways people live on this globe, and the variety of landscapes and land formations, or how volcanos or earthquakes work. I hope you can tell that I enjoy learning!
Homeschooling Carnival
This week the Carnival of Homescooling is held at palmtreepundit.blogspot.com. There were several math-related posts (including mine), again! I so delighted in reading and realizing that homeschoolers are appreciating mathematics and treating it 'positively'.
This is so important, as I've stressed many times. Our attitudes towards math (or history!) influence our students. An enthusiastic teacher is a big part of learning to enjoy a subject.
I am sincerely looking forward to re-learning geography, for example, with my kids. Here's a pick from the carnival: seems like US youngsters do not know geography very well... I think it is a fascinating subject, to learn about all the variety in the ways people live on this globe, and the variety of landscapes and land formations, or how volcanos or earthquakes work. I hope you can tell that I enjoy learning!
This is so important, as I've stressed many times. Our attitudes towards math (or history!) influence our students. An enthusiastic teacher is a big part of learning to enjoy a subject.
I am sincerely looking forward to re-learning geography, for example, with my kids. Here's a pick from the carnival: seems like US youngsters do not know geography very well... I think it is a fascinating subject, to learn about all the variety in the ways people live on this globe, and the variety of landscapes and land formations, or how volcanos or earthquakes work. I hope you can tell that I enjoy learning!
Math ebooks summer sale
I'm having a summer sale on my elementary math ebooks... They are not overpriced even normally and now you get some good discounts. Valid till end of July, I offer:
These ebooks cover topics in grades 1-5, such as addition facts, multiplication tables, division, long division, factoring, fractions, decimals, and geometry. They are 'worktexts' with explanations and problems in the same product. The individual ebooks start from $1.50.
Learn more about the ebooks and see preview pages.
Tags: math
- the 18 ebooks package for $21.90 (before $27.90)!
- the 18 ebooks package on CD for $27.90 (before $33.90)!
- order totals at least $10 - 20% discount
- order totals between $15.10 and $21 - 30% discount
These ebooks cover topics in grades 1-5, such as addition facts, multiplication tables, division, long division, factoring, fractions, decimals, and geometry. They are 'worktexts' with explanations and problems in the same product. The individual ebooks start from $1.50.
Learn more about the ebooks and see preview pages.
Tags: math
Math ebooks summer sale
I'm having a summer sale on my elementary math ebooks... They are not overpriced even normally and now you get some good discounts. Valid till end of July, I offer:
These ebooks cover topics in grades 1-5, such as addition facts, multiplication tables, division, long division, factoring, fractions, decimals, and geometry. They are 'worktexts' with explanations and problems in the same product. The individual ebooks start from $1.50.
Learn more about the ebooks and see preview pages.
Tags: math
- the 18 ebooks package for $21.90 (before $27.90)!
- the 18 ebooks package on CD for $27.90 (before $33.90)!
- order totals at least $10 - 20% discount
- order totals between $15.10 and $21 - 30% discount
These ebooks cover topics in grades 1-5, such as addition facts, multiplication tables, division, long division, factoring, fractions, decimals, and geometry. They are 'worktexts' with explanations and problems in the same product. The individual ebooks start from $1.50.
Learn more about the ebooks and see preview pages.
Tags: math
Monday, June 5, 2006
Coming soon...
I'm planning on a SUMMER DISCOUNT on my ebooks package. It currently sells for $27.90, but I will soon lower the price for summer to $21.90.
There are 18 ebooks, covering topics from basic addition concept, mental math, subtraction, multiplication, times tables, division, place value, fractions, and basics of decimals.
They are worktexts, with explanations and problems. All total the ebooks have over 770 pages of instruction. That makes it... 2.8 cents per page.
You simply download the ebook, and print out the pages you want - and you can print them out over and over again if needed.
Just stay tuned... I will announce the actual summer sale soon. Meanwhile, feel free to browse the preview pages at HomeschoolMath.net/ebooks.php.
There are 18 ebooks, covering topics from basic addition concept, mental math, subtraction, multiplication, times tables, division, place value, fractions, and basics of decimals.
They are worktexts, with explanations and problems. All total the ebooks have over 770 pages of instruction. That makes it... 2.8 cents per page.
You simply download the ebook, and print out the pages you want - and you can print them out over and over again if needed.
Just stay tuned... I will announce the actual summer sale soon. Meanwhile, feel free to browse the preview pages at HomeschoolMath.net/ebooks.php.
Coming soon...
I'm planning on a SUMMER DISCOUNT on my ebooks package. It currently sells for $27.90, but I will soon lower the price for summer to $21.90.
There are 18 ebooks, covering topics from basic addition concept, mental math, subtraction, multiplication, times tables, division, place value, fractions, and basics of decimals.
They are worktexts, with explanations and problems. All total the ebooks have over 770 pages of instruction. That makes it... 2.8 cents per page.
You simply download the ebook, and print out the pages you want - and you can print them out over and over again if needed.
Just stay tuned... I will announce the actual summer sale soon. Meanwhile, feel free to browse the preview pages at HomeschoolMath.net/ebooks.php.
There are 18 ebooks, covering topics from basic addition concept, mental math, subtraction, multiplication, times tables, division, place value, fractions, and basics of decimals.
They are worktexts, with explanations and problems. All total the ebooks have over 770 pages of instruction. That makes it... 2.8 cents per page.
You simply download the ebook, and print out the pages you want - and you can print them out over and over again if needed.
Just stay tuned... I will announce the actual summer sale soon. Meanwhile, feel free to browse the preview pages at HomeschoolMath.net/ebooks.php.
Sunday, June 4, 2006
What jobs use Pythagorean theorem?
This is what someone asked me recently.
Well, obviously you'd need to know Pythagorean theorem if your job includes triangles or rectangles in some way, for example in land surveying, or planning farmland, or designing buildings (architects) or designing furniture or frames for TVs, or making parts for machines (machinist) etc.
Remember the diagonal for a rectangle is calculated using Pythagorean theorem, and TV screen size is actually the length of the diagonal in inches.
See also my article on similar lines: Where do you need square roots or algebra - why study math?.
Well, obviously you'd need to know Pythagorean theorem if your job includes triangles or rectangles in some way, for example in land surveying, or planning farmland, or designing buildings (architects) or designing furniture or frames for TVs, or making parts for machines (machinist) etc.
Remember the diagonal for a rectangle is calculated using Pythagorean theorem, and TV screen size is actually the length of the diagonal in inches.
See also my article on similar lines: Where do you need square roots or algebra - why study math?.
What jobs use Pythagorean theorem?
This is what someone asked me recently.
Well, obviously you'd need to know Pythagorean theorem if your job includes triangles or rectangles in some way, for example in land surveying, or planning farmland, or designing buildings (architects) or designing furniture or frames for TVs, or making parts for machines (machinist) etc.
Remember the diagonal for a rectangle is calculated using Pythagorean theorem, and TV screen size is actually the length of the diagonal in inches.
See also my article on similar lines: Where do you need square roots or algebra - why study math?.
Well, obviously you'd need to know Pythagorean theorem if your job includes triangles or rectangles in some way, for example in land surveying, or planning farmland, or designing buildings (architects) or designing furniture or frames for TVs, or making parts for machines (machinist) etc.
Remember the diagonal for a rectangle is calculated using Pythagorean theorem, and TV screen size is actually the length of the diagonal in inches.
See also my article on similar lines: Where do you need square roots or algebra - why study math?.
Friday, June 2, 2006
Sperm Whale
The sperm whale is the largest breed of toothed whale, it is distinctively characterized by its large head and can be one-third of its body. It also has a small lower jaw with conical teeth which fit into sockets on its upper jaw, a white mouth with a bright red tongue. They where named after their sought after "spermaceti oil" produced by their spermaceti organ located on its head. One look at the sperm whale and you might recognized it as the beast immortalized by Herman Melville in Moby Dick. This paper model was designed by Ryo Tokisato (Japan).






Sperm Whale - Download






Sperm Whale - Download
10 Out - math card game
Today I told my dd to write down addition facts where the sum is 10 - "Which numbers make ten?" are the words I use with her. While she was writing them and using abacus, this math card came 'popped' to my mind...
I can't claim it as my own, because I have this 'feeling' that I've read about it somewhere, sometime, but since I can't remember when or where, I can't give credit to where credit might be due.
I will just name it "10 Out" - a math card game.
Anyway, this is how it goes:
Take away the 'picture' cards and joker from normal playing cards. Then deal 10 cards to each player; put the rest of the deck in the middle. The goal is to get rid of all cards in your hand.
Find all pairs of cards in your hand that add up to a ten (or single 10's) and discard them. Then you may ask the player left to you for one card, and if she has it, she has to give it to you.
For example, say you have 2, 3, 8, and 1 left in your hand. You ask the person next to you if he has 9 (because 9 and 1 would make ten). He has, and gives it to you. Then you form another ten and discard your 1 and the 9.
Then the next person asks the player to their left for one card, and on around.
You could modify it so that each players just blindly hands one card to the player on their left.
Then, once nobody can discard any more cards, every player takes one card from the deck in the middle, and checks if they can form a sum of ten and discard cards. Again each player will be allowed to ask for one card from their left neighbor, if they want to (or everyone hands one card blindly to the player on their left). Continue until someone wins.
I feel this can easily make kids memorize addition facts with sum 10.
Then, of course, next math lesson it will be time for "9 Out" and "8 Out" and "11 Out" and others!
Tags: math
I can't claim it as my own, because I have this 'feeling' that I've read about it somewhere, sometime, but since I can't remember when or where, I can't give credit to where credit might be due.
I will just name it "10 Out" - a math card game.
Anyway, this is how it goes:
Take away the 'picture' cards and joker from normal playing cards. Then deal 10 cards to each player; put the rest of the deck in the middle. The goal is to get rid of all cards in your hand.
Find all pairs of cards in your hand that add up to a ten (or single 10's) and discard them. Then you may ask the player left to you for one card, and if she has it, she has to give it to you.
For example, say you have 2, 3, 8, and 1 left in your hand. You ask the person next to you if he has 9 (because 9 and 1 would make ten). He has, and gives it to you. Then you form another ten and discard your 1 and the 9.
Then the next person asks the player to their left for one card, and on around.
You could modify it so that each players just blindly hands one card to the player on their left.
Then, once nobody can discard any more cards, every player takes one card from the deck in the middle, and checks if they can form a sum of ten and discard cards. Again each player will be allowed to ask for one card from their left neighbor, if they want to (or everyone hands one card blindly to the player on their left). Continue until someone wins.
I feel this can easily make kids memorize addition facts with sum 10.
Then, of course, next math lesson it will be time for "9 Out" and "8 Out" and "11 Out" and others!
Tags: math
10 Out - math card game
Today I told my dd to write down addition facts where the sum is 10 - "Which numbers make ten?" are the words I use with her. While she was writing them and using abacus, this math card came 'popped' to my mind...
I can't claim it as my own, because I have this 'feeling' that I've read about it somewhere, sometime, but since I can't remember when or where, I can't give credit to where credit might be due.
I will just name it "10 Out" - a math card game.
Anyway, this is how it goes:
Take away the 'picture' cards and joker from normal playing cards. Then deal 10 cards to each player; put the rest of the deck in the middle. The goal is to get rid of all cards in your hand.
Find all pairs of cards in your hand that add up to a ten (or single 10's) and discard them. Then you may ask the player left to you for one card, and if she has it, she has to give it to you.
For example, say you have 2, 3, 8, and 1 left in your hand. You ask the person next to you if he has 9 (because 9 and 1 would make ten). He has, and gives it to you. Then you form another ten and discard your 1 and the 9.
Then the next person asks the player to their left for one card, and on around.
You could modify it so that each players just blindly hands one card to the player on their left.
Then, once nobody can discard any more cards, every player takes one card from the deck in the middle, and checks if they can form a sum of ten and discard cards. Again each player will be allowed to ask for one card from their left neighbor, if they want to (or everyone hands one card blindly to the player on their left). Continue until someone wins.
I feel this can easily make kids memorize addition facts with sum 10.
Then, of course, next math lesson it will be time for "9 Out" and "8 Out" and "11 Out" and others!
Tags: math
I can't claim it as my own, because I have this 'feeling' that I've read about it somewhere, sometime, but since I can't remember when or where, I can't give credit to where credit might be due.
I will just name it "10 Out" - a math card game.
Anyway, this is how it goes:
Take away the 'picture' cards and joker from normal playing cards. Then deal 10 cards to each player; put the rest of the deck in the middle. The goal is to get rid of all cards in your hand.
Find all pairs of cards in your hand that add up to a ten (or single 10's) and discard them. Then you may ask the player left to you for one card, and if she has it, she has to give it to you.
For example, say you have 2, 3, 8, and 1 left in your hand. You ask the person next to you if he has 9 (because 9 and 1 would make ten). He has, and gives it to you. Then you form another ten and discard your 1 and the 9.
Then the next person asks the player to their left for one card, and on around.
You could modify it so that each players just blindly hands one card to the player on their left.
Then, once nobody can discard any more cards, every player takes one card from the deck in the middle, and checks if they can form a sum of ten and discard cards. Again each player will be allowed to ask for one card from their left neighbor, if they want to (or everyone hands one card blindly to the player on their left). Continue until someone wins.
I feel this can easily make kids memorize addition facts with sum 10.
Then, of course, next math lesson it will be time for "9 Out" and "8 Out" and "11 Out" and others!
Tags: math
Subscribe to:
Posts (Atom)
