My child is dealing identifying Triangles as SAS,SSS,AAS,ASA and HL Theroms. She is also having trouble with the Flow proofs and Column proofs on explaing why 2 triangles are congruent.
All of the theorems about proving that triangles (or other shapes) are congruent can be "translated" into a drawing problem:
If I have my 'secret' triangle and I give you THESE pieces of information, can you reproduce my triangle? Can you do that every time, no matter what my triangle?
We can describe a triangle using 6 pieces of information: the legths of the three sides, and the measures of the three angles. But you don't need all of those to be able to draw my secret triangle.
Can you draw a copy of my triangle if I tell you that....
- my triangle has a side 5 cm long, another side 6 cm long, and the angle between those sides is 29 degrees (I've given you S - A - S)?
- my triangle has a 30 degree angle, a 60 degree angle, and a 90 degree angle (I've given you A - A - A)?
In the first case, yes, and in the second case, no.
On proving problems, often the two triangles in the figure look obviously congruent and so it may be hard to see what there is to prove. But you simply need to look for corresponding side and angle parts in the two triangles that are known to be the same length or same angle measure.
Two angles in a figure might be the same if they're vertical angles or corresponding angles, or base angles of a isosceles triangle. (Or it is given.)
Two sides might be the same length if they are halves of a longer line (there is a midpoint). Or, they ARE the same line. Or you have a rectangle or square somewhere. (Or, it is given.)
For example, the image below does NOT depict two congruent triangles.
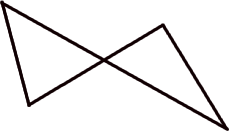
But this image does - because I made the point K to be a midpoint for the two intersecting line segments.
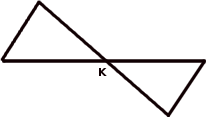
So there you have two pairs of lines of same length. And you have vertical angles which are the same - can you see how we then have S - A - S same in the two triangles -> they're congruent.
Maybe it would help to read explanations of this stuff a few times, from different places, with different wordings. It is not really difficult, I don't think!
Practice problems for congruent triangles from Yahoo education
Geometry: Congruent Triangles. Math for Morons like us.
Recognizing congruent triangles - interactive practice page from Oswego City School District Regents Exam Prep Center
Proving Rriangles Congruent interactive gizmo from ExploreLearning.com.
- you get 5 minutes free exploration time. It's a great site and great 'gizmos'.
What is proof - my own article.
Tags: math, mathematics, geometry, triangles, proof

No comments:
Post a Comment