I'm not a big fan of these various math tricks (such as how to multiply any two numbers between 11 and 19 or how to square some special numbers ending in certain digits) unless they increase students' understanding of the number system or math principles. Often they're just another thing to memorize that fades away quickly... at least with me.
But some people like them, and that's fine with me.
One particular kind of math "trick" is where you are told to think of a number, then do all sorts of manipulations with it, and then in the end... they know your number! How is that?
Here's one for you... it's neat, easy, amusing, and explains in the end how it works.
Scroll Down Slowly!
Anime, movie, comic book, video game, or TV related papercrafts, paper models and paper toys.
Wednesday, May 31, 2006
I can guess your thoughts trick
I'm not a big fan of these various math tricks (such as how to multiply any two numbers between 11 and 19 or how to square some special numbers ending in certain digits) unless they increase students' understanding of the number system or math principles. Often they're just another thing to memorize that fades away quickly... at least with me.
But some people like them, and that's fine with me.
One particular kind of math "trick" is where you are told to think of a number, then do all sorts of manipulations with it, and then in the end... they know your number! How is that?
Here's one for you... it's neat, easy, amusing, and explains in the end how it works.
Scroll Down Slowly!
But some people like them, and that's fine with me.
One particular kind of math "trick" is where you are told to think of a number, then do all sorts of manipulations with it, and then in the end... they know your number! How is that?
Here's one for you... it's neat, easy, amusing, and explains in the end how it works.
Scroll Down Slowly!
Tuesday, May 30, 2006
Math card game
Last few weeks I've been thinking I want to have a way to use normal playing cards to let my daughter practice addition.
I hadn't given it serious 'thought' to come up with any games yet, when just today I stumbled upon that exact thing at A Home for Homeschoolers Portal: The game that is worth 1,000 worksheets.
Definitely worth playing! The 'game' aspect can make practicing facts so much more fun, as we all know. And this game that Denise describes is so simple too.
If you have any other playing card ideas, feel free to comment here or there.
I hadn't given it serious 'thought' to come up with any games yet, when just today I stumbled upon that exact thing at A Home for Homeschoolers Portal: The game that is worth 1,000 worksheets.
Definitely worth playing! The 'game' aspect can make practicing facts so much more fun, as we all know. And this game that Denise describes is so simple too.
If you have any other playing card ideas, feel free to comment here or there.
Math card game
Last few weeks I've been thinking I want to have a way to use normal playing cards to let my daughter practice addition.
I hadn't given it serious 'thought' to come up with any games yet, when just today I stumbled upon that exact thing at A Home for Homeschoolers Portal: The game that is worth 1,000 worksheets.
Definitely worth playing! The 'game' aspect can make practicing facts so much more fun, as we all know. And this game that Denise describes is so simple too.
If you have any other playing card ideas, feel free to comment here or there.
I hadn't given it serious 'thought' to come up with any games yet, when just today I stumbled upon that exact thing at A Home for Homeschoolers Portal: The game that is worth 1,000 worksheets.
Definitely worth playing! The 'game' aspect can make practicing facts so much more fun, as we all know. And this game that Denise describes is so simple too.
If you have any other playing card ideas, feel free to comment here or there.
Saturday, May 27, 2006
The exponential function
I just finished reading the third volume of Calculus Without Tears... worksheets designed to teach calculus concepts before high school, for those interested.
So I've gotten a refresher on calculus... which I've always loved, and so that's why in today's post I hope to explain to you ONE little point from calculus - a fascinating and important one - that is.... THE EXPONENTIAL FUNCTION and THE NUMBER E.
First you need to know a calculus concept, and something about e:
Simple enough, huh?
Okay, so here we go:
There is a function....
whose growth rate at any point
is always
its value at that point!

This is a remarkable property, and ex is the ONLY function with this property: that its growth rate at any point is the same as its value at that point.
But there is more:
You know how sine and cosine are 'waves' when their graphs are plotted. They don't look anything like the exponential function, which just shoots straight up!
BUT when you switch to the complex numbers, they all three are deeply connected with each other:
Isn't that something showing us the importance of the exponential function?
Then, who would have guessed that if you look at the sequence...
1 + 1/1
(1 + 1/2)2
(1 + 1/3)3
(1 + 1/4)4
(1 + 1/5)5
(1 + 1/6)6
(1 + 1/7)7
(1 + 1/8)8
(1 + 1/9)9.
.
.
.
...that it tends to e! (In other words, its limit is e as n → infinity.)
Check for yourself by using this nice interactive thing where you slide the sliding bar to change value of n.
This number e shows up everywhere!!! No wonder it is often compared to Pi, and those two together are like "GIANTS" or "biggies" when it comes to special numbers.
I hope your head is not spinning too fast (if you've never studied calculus before). I just wanted to show some 'glimpses' of the 'goodies' of calculus... it is fascinating. It's like anywhere mathematicians turn, they find Pi and e.... those two immensely important irrational numbers ... lots of connections in different areas of mathematics - and I don't know but a tiny fraction of those. All of it is beyond me... I stand in awe...
Tags: math, mathematics, calculus
So I've gotten a refresher on calculus... which I've always loved, and so that's why in today's post I hope to explain to you ONE little point from calculus - a fascinating and important one - that is.... THE EXPONENTIAL FUNCTION and THE NUMBER E.
First you need to know a calculus concept, and something about e:
- The steeper the graph of a function, the faster it's growing (and vice versa)
- We can measure the steepness of a function at any point by drawing a tangent, and checking the slope of the tangent.
- e is a VERY special irrational number with the approximate value 2.718281828459...
Simple enough, huh?
Okay, so here we go:
There is a function....
whose growth rate at any point
is always
its value at that point!

The function ex

The 'steepness' of the tangent shows us the growth rate of the function.
Tangent drawn at x=0 has slope 1 - which is the value of ex when x=0.

Here is a tangent to ex drawn at x=1.

Zooming in closer... The tangent drawn at x=1 has slope 2.718... - which is the value of ex at x=1.

The 'steepness' of the tangent shows us the growth rate of the function.
Tangent drawn at x=0 has slope 1 - which is the value of ex when x=0.

Here is a tangent to ex drawn at x=1.

Zooming in closer... The tangent drawn at x=1 has slope 2.718... - which is the value of ex at x=1.
This is a remarkable property, and ex is the ONLY function with this property: that its growth rate at any point is the same as its value at that point.
But there is more:
You know how sine and cosine are 'waves' when their graphs are plotted. They don't look anything like the exponential function, which just shoots straight up!
BUT when you switch to the complex numbers, they all three are deeply connected with each other:
eix = cos x + i sin x
(Euler's formula - e also gets its name from Euler)
ei&pi + 1 = 0
A special case of Euler's formula, linking five important numbers e, i, π, 1, and 0.
cos x = (eix + e-ix)/2
sin x = (eix - e-ix)/2i
These two expressions show that both cosine and sine can be written using exponential function alone.
(Euler's formula - e also gets its name from Euler)
ei&pi + 1 = 0
A special case of Euler's formula, linking five important numbers e, i, π, 1, and 0.
cos x = (eix + e-ix)/2
sin x = (eix - e-ix)/2i
These two expressions show that both cosine and sine can be written using exponential function alone.
Isn't that something showing us the importance of the exponential function?
Then, who would have guessed that if you look at the sequence...
1 + 1/1
(1 + 1/2)2
(1 + 1/3)3
(1 + 1/4)4
(1 + 1/5)5
(1 + 1/6)6
(1 + 1/7)7
(1 + 1/8)8
(1 + 1/9)9.
.
.
.
...that it tends to e! (In other words, its limit is e as n → infinity.)
Check for yourself by using this nice interactive thing where you slide the sliding bar to change value of n.
This number e shows up everywhere!!! No wonder it is often compared to Pi, and those two together are like "GIANTS" or "biggies" when it comes to special numbers.
With the possible exception of pi, e is the most important constant in mathematics since it appears in myriad mathematical contexts involving limits and derivatives.
(http://mathworld.wolfram.com/e.html)
As far as there being other nice numbers that come up all the time, e and Pi are certainly the two biggies. There's another number, called the golden ratio, which is (1+√5)/2. It doesn't look all that nice at first glance, but it has some nice properties too, and the Greeks liked it a lot. But it doesn't come up nearly as much as e or Pi, so I guess it's not on par with the giants.
Why is e so important - Ask Dr. Math®
I hope your head is not spinning too fast (if you've never studied calculus before). I just wanted to show some 'glimpses' of the 'goodies' of calculus... it is fascinating. It's like anywhere mathematicians turn, they find Pi and e.... those two immensely important irrational numbers ... lots of connections in different areas of mathematics - and I don't know but a tiny fraction of those. All of it is beyond me... I stand in awe...
Tags: math, mathematics, calculus
The exponential function
I just finished reading the third volume of Calculus Without Tears... worksheets designed to teach calculus concepts before high school, for those interested.
So I've gotten a refresher on calculus... which I've always loved, and so that's why in today's post I hope to explain to you ONE little point from calculus - a fascinating and important one - that is.... THE EXPONENTIAL FUNCTION and THE NUMBER E.
First you need to know a calculus concept, and something about e:
Simple enough, huh?
Okay, so here we go:
There is a function....
whose growth rate at any point
is always
its value at that point!

This is a remarkable property, and ex is the ONLY function with this property: that its growth rate at any point is the same as its value at that point.
But there is more:
You know how sine and cosine are 'waves' when their graphs are plotted. They don't look anything like the exponential function, which just shoots straight up!
BUT when you switch to the complex numbers, they all three are deeply connected with each other:
Isn't that something showing us the importance of the exponential function?
Then, who would have guessed that if you look at the sequence...
1 + 1/1
(1 + 1/2)2
(1 + 1/3)3
(1 + 1/4)4
(1 + 1/5)5
(1 + 1/6)6
(1 + 1/7)7
(1 + 1/8)8
(1 + 1/9)9.
.
.
.
...that it tends to e! (In other words, its limit is e as n → infinity.)
Check for yourself by using this nice interactive thing where you slide the sliding bar to change value of n.
This number e shows up everywhere!!! No wonder it is often compared to Pi, and those two together are like "GIANTS" or "biggies" when it comes to special numbers.
I hope your head is not spinning too fast (if you've never studied calculus before). I just wanted to show some 'glimpses' of the 'goodies' of calculus... it is fascinating. It's like anywhere mathematicians turn, they find Pi and e.... those two immensely important irrational numbers ... lots of connections in different areas of mathematics - and I don't know but a tiny fraction of those. All of it is beyond me... I stand in awe...
Tags: math, mathematics, calculus
So I've gotten a refresher on calculus... which I've always loved, and so that's why in today's post I hope to explain to you ONE little point from calculus - a fascinating and important one - that is.... THE EXPONENTIAL FUNCTION and THE NUMBER E.
First you need to know a calculus concept, and something about e:
- The steeper the graph of a function, the faster it's growing (and vice versa)
- We can measure the steepness of a function at any point by drawing a tangent, and checking the slope of the tangent.
- e is a VERY special irrational number with the approximate value 2.718281828459...
Simple enough, huh?
Okay, so here we go:
There is a function....
whose growth rate at any point
is always
its value at that point!

The function ex

The 'steepness' of the tangent shows us the growth rate of the function.
Tangent drawn at x=0 has slope 1 - which is the value of ex when x=0.

Here is a tangent to ex drawn at x=1.

Zooming in closer... The tangent drawn at x=1 has slope 2.718... - which is the value of ex at x=1.

The 'steepness' of the tangent shows us the growth rate of the function.
Tangent drawn at x=0 has slope 1 - which is the value of ex when x=0.

Here is a tangent to ex drawn at x=1.

Zooming in closer... The tangent drawn at x=1 has slope 2.718... - which is the value of ex at x=1.
This is a remarkable property, and ex is the ONLY function with this property: that its growth rate at any point is the same as its value at that point.
But there is more:
You know how sine and cosine are 'waves' when their graphs are plotted. They don't look anything like the exponential function, which just shoots straight up!
BUT when you switch to the complex numbers, they all three are deeply connected with each other:
eix = cos x + i sin x
(Euler's formula - e also gets its name from Euler)
ei&pi + 1 = 0
A special case of Euler's formula, linking five important numbers e, i, π, 1, and 0.
cos x = (eix + e-ix)/2
sin x = (eix - e-ix)/2i
These two expressions show that both cosine and sine can be written using exponential function alone.
(Euler's formula - e also gets its name from Euler)
ei&pi + 1 = 0
A special case of Euler's formula, linking five important numbers e, i, π, 1, and 0.
cos x = (eix + e-ix)/2
sin x = (eix - e-ix)/2i
These two expressions show that both cosine and sine can be written using exponential function alone.
Isn't that something showing us the importance of the exponential function?
Then, who would have guessed that if you look at the sequence...
1 + 1/1
(1 + 1/2)2
(1 + 1/3)3
(1 + 1/4)4
(1 + 1/5)5
(1 + 1/6)6
(1 + 1/7)7
(1 + 1/8)8
(1 + 1/9)9.
.
.
.
...that it tends to e! (In other words, its limit is e as n → infinity.)
Check for yourself by using this nice interactive thing where you slide the sliding bar to change value of n.
This number e shows up everywhere!!! No wonder it is often compared to Pi, and those two together are like "GIANTS" or "biggies" when it comes to special numbers.
With the possible exception of pi, e is the most important constant in mathematics since it appears in myriad mathematical contexts involving limits and derivatives.
(http://mathworld.wolfram.com/e.html)
As far as there being other nice numbers that come up all the time, e and Pi are certainly the two biggies. There's another number, called the golden ratio, which is (1+√5)/2. It doesn't look all that nice at first glance, but it has some nice properties too, and the Greeks liked it a lot. But it doesn't come up nearly as much as e or Pi, so I guess it's not on par with the giants.
Why is e so important - Ask Dr. Math®
I hope your head is not spinning too fast (if you've never studied calculus before). I just wanted to show some 'glimpses' of the 'goodies' of calculus... it is fascinating. It's like anywhere mathematicians turn, they find Pi and e.... those two immensely important irrational numbers ... lots of connections in different areas of mathematics - and I don't know but a tiny fraction of those. All of it is beyond me... I stand in awe...
Tags: math, mathematics, calculus
Friday, May 26, 2006
Green Dragon Papercraft
Here's another cute paper model from Ryo Tokisato, a cute little green dragon. It's pretty easy to build and a good activity for the young and young-at-heart to do this Memorial Day weekend if your staying indoors. It's a single PDF file with both the pattern and instructions in it. Have fun!






Green Dragon Papercraft [Ryo Tokisato]






Green Dragon Papercraft [Ryo Tokisato]
Wednesday, May 24, 2006
Gojimon
Received lots of request this week from parents for simple and easy to do paper models that they could do with their kids. So this next few weeks we are going to feature the work of Ryo Tokisato, he has a collection of paper models that are fun, quirky, kid-friendly, and most of all easy to do. This first paper model that we are going to feature is Gojimon, he is a funny looking green dragon. Gojimon is in a single PDF file with both the paper pattern and instructions.






Gojimon - Download






Gojimon - Download
Website updates
I seem to get so involved in writing about math topics that I forget that I'm supposed to also write about my website updates. So here goes, some recent updates and additions:
1) I've reorganized the links and stuff on the reviews page and on the math curriculum reviews page, for easier navigating. (Not that my site couldn't use more improvement in that navigation... hopefully some day.)
2) Added a page for Thinkwell CDs. If you've used those, please leave a review.
3) I made the blogposts and discussions a few months back about word problems into an article: Teaching elementary word problems.
4) This month I've had some new advertisers:
I guess that's all that I can remember. Have a good day!
1) I've reorganized the links and stuff on the reviews page and on the math curriculum reviews page, for easier navigating. (Not that my site couldn't use more improvement in that navigation... hopefully some day.)
2) Added a page for Thinkwell CDs. If you've used those, please leave a review.
3) I made the blogposts and discussions a few months back about word problems into an article: Teaching elementary word problems.
4) This month I've had some new advertisers:
- Maths2xl.co.uk - individual audio, animated, secondary maths lessons with accompanying worksheets and automatic marking.
- MathScore.com - unlimited online math practice
- Time4Learning.com - an online curriculum with animated lessons for $19.99 per month. There is more info and reviews here.
I guess that's all that I can remember. Have a good day!
Website updates
I seem to get so involved in writing about math topics that I forget that I'm supposed to also write about my website updates. So here goes, some recent updates and additions:
1) I've reorganized the links and stuff on the reviews page and on the math curriculum reviews page, for easier navigating. (Not that my site couldn't use more improvement in that navigation... hopefully some day.)
2) Added a page for Thinkwell CDs. If you've used those, please leave a review.
3) I made the blogposts and discussions a few months back about word problems into an article: Teaching elementary word problems.
4) This month I've had some new advertisers:
I guess that's all that I can remember. Have a good day!
1) I've reorganized the links and stuff on the reviews page and on the math curriculum reviews page, for easier navigating. (Not that my site couldn't use more improvement in that navigation... hopefully some day.)
2) Added a page for Thinkwell CDs. If you've used those, please leave a review.
3) I made the blogposts and discussions a few months back about word problems into an article: Teaching elementary word problems.
4) This month I've had some new advertisers:
- Maths2xl.co.uk - individual audio, animated, secondary maths lessons with accompanying worksheets and automatic marking.
- MathScore.com - unlimited online math practice
- Time4Learning.com - an online curriculum with animated lessons for $19.99 per month. There is more info and reviews here.
I guess that's all that I can remember. Have a good day!
Monday, May 22, 2006
Proving triangles congruent
My child is dealing identifying Triangles as SAS,SSS,AAS,ASA and HL Theroms. She is also having trouble with the Flow proofs and Column proofs on explaing why 2 triangles are congruent.
All of the theorems about proving that triangles (or other shapes) are congruent can be "translated" into a drawing problem:
If I have my 'secret' triangle and I give you THESE pieces of information, can you reproduce my triangle? Can you do that every time, no matter what my triangle?
We can describe a triangle using 6 pieces of information: the legths of the three sides, and the measures of the three angles. But you don't need all of those to be able to draw my secret triangle.
Can you draw a copy of my triangle if I tell you that....
- my triangle has a side 5 cm long, another side 6 cm long, and the angle between those sides is 29 degrees (I've given you S - A - S)?
- my triangle has a 30 degree angle, a 60 degree angle, and a 90 degree angle (I've given you A - A - A)?
In the first case, yes, and in the second case, no.
On proving problems, often the two triangles in the figure look obviously congruent and so it may be hard to see what there is to prove. But you simply need to look for corresponding side and angle parts in the two triangles that are known to be the same length or same angle measure.
Two angles in a figure might be the same if they're vertical angles or corresponding angles, or base angles of a isosceles triangle. (Or it is given.)
Two sides might be the same length if they are halves of a longer line (there is a midpoint). Or, they ARE the same line. Or you have a rectangle or square somewhere. (Or, it is given.)
For example, the image below does NOT depict two congruent triangles.
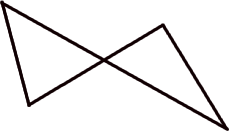
But this image does - because I made the point K to be a midpoint for the two intersecting line segments.
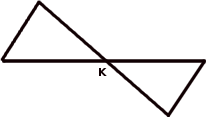
So there you have two pairs of lines of same length. And you have vertical angles which are the same - can you see how we then have S - A - S same in the two triangles -> they're congruent.
Maybe it would help to read explanations of this stuff a few times, from different places, with different wordings. It is not really difficult, I don't think!
Practice problems for congruent triangles from Yahoo education
Geometry: Congruent Triangles. Math for Morons like us.
Recognizing congruent triangles - interactive practice page from Oswego City School District Regents Exam Prep Center
Proving Rriangles Congruent interactive gizmo from ExploreLearning.com.
- you get 5 minutes free exploration time. It's a great site and great 'gizmos'.
What is proof - my own article.
Tags: math, mathematics, geometry, triangles, proof
Proving triangles congruent
My child is dealing identifying Triangles as SAS,SSS,AAS,ASA and HL Theroms. She is also having trouble with the Flow proofs and Column proofs on explaing why 2 triangles are congruent.
All of the theorems about proving that triangles (or other shapes) are congruent can be "translated" into a drawing problem:
If I have my 'secret' triangle and I give you THESE pieces of information, can you reproduce my triangle? Can you do that every time, no matter what my triangle?
We can describe a triangle using 6 pieces of information: the legths of the three sides, and the measures of the three angles. But you don't need all of those to be able to draw my secret triangle.
Can you draw a copy of my triangle if I tell you that....
- my triangle has a side 5 cm long, another side 6 cm long, and the angle between those sides is 29 degrees (I've given you S - A - S)?
- my triangle has a 30 degree angle, a 60 degree angle, and a 90 degree angle (I've given you A - A - A)?
In the first case, yes, and in the second case, no.
On proving problems, often the two triangles in the figure look obviously congruent and so it may be hard to see what there is to prove. But you simply need to look for corresponding side and angle parts in the two triangles that are known to be the same length or same angle measure.
Two angles in a figure might be the same if they're vertical angles or corresponding angles, or base angles of a isosceles triangle. (Or it is given.)
Two sides might be the same length if they are halves of a longer line (there is a midpoint). Or, they ARE the same line. Or you have a rectangle or square somewhere. (Or, it is given.)
For example, the image below does NOT depict two congruent triangles.

But this image does - because I made the point K to be a midpoint for the two intersecting line segments.

So there you have two pairs of lines of same length. And you have vertical angles which are the same - can you see how we then have S - A - S same in the two triangles -> they're congruent.
Maybe it would help to read explanations of this stuff a few times, from different places, with different wordings. It is not really difficult, I don't think!
Practice problems for congruent triangles from Yahoo education
Geometry: Congruent Triangles. Math for Morons like us.
Recognizing congruent triangles - interactive practice page from Oswego City School District Regents Exam Prep Center
Proving Rriangles Congruent interactive gizmo from ExploreLearning.com.
- you get 5 minutes free exploration time. It's a great site and great 'gizmos'.
What is proof - my own article.
Tags: math, mathematics, geometry, triangles, proof
Thursday, May 18, 2006
Count On, Relax, Enjoy
Summer is approaching, and you all my readers probably have all kinds of different plans. If you're a homeschooler, maybe you continue homeschool, maybe not. Some people take a vacation, some stay at home.
Anyway, however it might fit into your plans, I'd like to recommend a nice (British) math website Count On (Counton.org).
In my mind, Count On website is almost like a mathematical vacation, full of enjoyment with mathematical themes. Even the logo looks like the site is supposed to make you feel happy.

It has various things on it: some very games of course, two interactive "museums" with galleries of mathematical paintings and explanations of their history (Matrix Museum and Pictures Interactive). A maths timeline for history geeks.
I especially like their "mathzines", or math related online magazines. There are two different ones: Meenie Minus for smaller kids, and Kaleidoscope for middle school age. The magazines have engaging, short games and activities, just like your printed kid magazines do.
The Explorer section is really neat, with activities on tilings, patterns, fractals, gridwarping, tricks, etc.
The Resources section has articles on math misconceptions, and some British curriculum guidelines etc.
And that wasn't all, but I stop here because I haven't even explored every corner of that site myself. I hope you can find some relaxing mathematical moments or fun activities for your students (OR YOURSELF). They even have a online maths newspaper called The Sum with quite some headlines!
Here's the link once again: Count On.
And no, this wasn't any paid advertisement or endorsement; the folks there know nothing about me blogging about their site... I just really appreciate their efforts in building the website and showing how math can be enjoyable.
Tags: math, mathematics
Anyway, however it might fit into your plans, I'd like to recommend a nice (British) math website Count On (Counton.org).
In my mind, Count On website is almost like a mathematical vacation, full of enjoyment with mathematical themes. Even the logo looks like the site is supposed to make you feel happy.

It has various things on it: some very games of course, two interactive "museums" with galleries of mathematical paintings and explanations of their history (Matrix Museum and Pictures Interactive). A maths timeline for history geeks.
I especially like their "mathzines", or math related online magazines. There are two different ones: Meenie Minus for smaller kids, and Kaleidoscope for middle school age. The magazines have engaging, short games and activities, just like your printed kid magazines do.
The Explorer section is really neat, with activities on tilings, patterns, fractals, gridwarping, tricks, etc.
The Resources section has articles on math misconceptions, and some British curriculum guidelines etc.
And that wasn't all, but I stop here because I haven't even explored every corner of that site myself. I hope you can find some relaxing mathematical moments or fun activities for your students (OR YOURSELF). They even have a online maths newspaper called The Sum with quite some headlines!
Here's the link once again: Count On.
And no, this wasn't any paid advertisement or endorsement; the folks there know nothing about me blogging about their site... I just really appreciate their efforts in building the website and showing how math can be enjoyable.
Tags: math, mathematics
Count On, Relax, Enjoy
Summer is approaching, and you all my readers probably have all kinds of different plans. If you're a homeschooler, maybe you continue homeschool, maybe not. Some people take a vacation, some stay at home.
Anyway, however it might fit into your plans, I'd like to recommend a nice (British) math website Count On (Counton.org).
In my mind, Count On website is almost like a mathematical vacation, full of enjoyment with mathematical themes. Even the logo looks like the site is supposed to make you feel happy.

It has various things on it: some very games of course, two interactive "museums" with galleries of mathematical paintings and explanations of their history (Matrix Museum and Pictures Interactive). A maths timeline for history geeks.
I especially like their "mathzines", or math related online magazines. There are two different ones: Meenie Minus for smaller kids, and Kaleidoscope for middle school age. The magazines have engaging, short games and activities, just like your printed kid magazines do.
The Explorer section is really neat, with activities on tilings, patterns, fractals, gridwarping, tricks, etc.
The Resources section has articles on math misconceptions, and some British curriculum guidelines etc.
And that wasn't all, but I stop here because I haven't even explored every corner of that site myself. I hope you can find some relaxing mathematical moments or fun activities for your students (OR YOURSELF). They even have a online maths newspaper called The Sum with quite some headlines!
Here's the link once again: Count On.
And no, this wasn't any paid advertisement or endorsement; the folks there know nothing about me blogging about their site... I just really appreciate their efforts in building the website and showing how math can be enjoyable.
Tags: math, mathematics
Anyway, however it might fit into your plans, I'd like to recommend a nice (British) math website Count On (Counton.org).
In my mind, Count On website is almost like a mathematical vacation, full of enjoyment with mathematical themes. Even the logo looks like the site is supposed to make you feel happy.

It has various things on it: some very games of course, two interactive "museums" with galleries of mathematical paintings and explanations of their history (Matrix Museum and Pictures Interactive). A maths timeline for history geeks.
I especially like their "mathzines", or math related online magazines. There are two different ones: Meenie Minus for smaller kids, and Kaleidoscope for middle school age. The magazines have engaging, short games and activities, just like your printed kid magazines do.
The Explorer section is really neat, with activities on tilings, patterns, fractals, gridwarping, tricks, etc.
The Resources section has articles on math misconceptions, and some British curriculum guidelines etc.
And that wasn't all, but I stop here because I haven't even explored every corner of that site myself. I hope you can find some relaxing mathematical moments or fun activities for your students (OR YOURSELF). They even have a online maths newspaper called The Sum with quite some headlines!
Here's the link once again: Count On.
And no, this wasn't any paid advertisement or endorsement; the folks there know nothing about me blogging about their site... I just really appreciate their efforts in building the website and showing how math can be enjoyable.
Tags: math, mathematics
Wednesday, May 17, 2006
Shadow the Hedgehog Papercraft
Now comes the second part to end E3 week, here is a paper model of Shadow the Hedgehog. To those who don't know much about Shadow, he is the rude, arrogant, destructive, and ultra-violent adversary to Sonic. Although that was the intention of his creator Yuji Uekawa, they softened up Shadows image when they brought him here to the US to show acceptability for a younger audience. One of Shadow's trademark move is the Chaos Control, in it, he is able to warp time and space and thereby allow himself to teleport in great distances. Shadow also has an alter-ego known as Super Shadow, in which he transform himself into a faster, stronger, and nearly invincible Shadow, but in a lemon-cream color instead of his usual black. Once again, the paper model was created by the Sega Sonicteam, the files are in JPG format and is zipped.






Shadow the Hedgehog [via MediaFire]
Sonic the Hedgehog [Related Post]






Shadow the Hedgehog [via MediaFire]
Sonic the Hedgehog [Related Post]
Carnival of Homeschooling
This week's carnival is online at Home Sweet Home. Melissa has arranged the contributions in an absolutely fascinating way - using animals and their characteristics! You have to go see it.
And, I also want to highlight a post I picked from there that fits well the theme of this blog: Number Bonds = Better Understanding.
And, I also want to highlight a post I picked from there that fits well the theme of this blog: Number Bonds = Better Understanding.
Carnival of Homeschooling
This week's carnival is online at Home Sweet Home. Melissa has arranged the contributions in an absolutely fascinating way - using animals and their characteristics! You have to go see it.
And, I also want to highlight a post I picked from there that fits well the theme of this blog: Number Bonds = Better Understanding.
And, I also want to highlight a post I picked from there that fits well the theme of this blog: Number Bonds = Better Understanding.
Monday, May 15, 2006
Missing addend with x or a box?
Recently I've been writing worksheets for fourth grade... Later this year, I will make this collection available for whoever wishes to buy it. But for now, it's a work in progress.
One topic I was pondering a lot was whether to include problems such as
15 + x = 30 into the worksheets.
Or, 234 + x = 700
or x + 1,923 = 5,000.
I hope you get the idea; in elementary books you often see these kind of missing addend problems with a little box:
15 + = 30 or
= 30 or
234 + = 700 or
= 700 or
 + 1,923 = 5,000.
+ 1,923 = 5,000.
Well, I decided for the x over the box! I don't think solving missing addend problems with subtraction is too difficult to learn on fourth grade; after all, students have been working with addition and subtraction connection from 1st grade on, right?
In my own old schoolbooks I actually see x from 3rd grade on.
I made several problems with charts such as
Hopefully those help the students with algebraic thinking.
Tags: math, elementary, algebra
One topic I was pondering a lot was whether to include problems such as
15 + x = 30 into the worksheets.
Or, 234 + x = 700
or x + 1,923 = 5,000.
I hope you get the idea; in elementary books you often see these kind of missing addend problems with a little box:
15 +
 = 30 or
= 30 or 234 +
 = 700 or
= 700 or + 1,923 = 5,000.
+ 1,923 = 5,000. Well, I decided for the x over the box! I don't think solving missing addend problems with subtraction is too difficult to learn on fourth grade; after all, students have been working with addition and subtraction connection from 1st grade on, right?
In my own old schoolbooks I actually see x from 3rd grade on.
I made several problems with charts such as
Write a missing addend sentence
and a subtraction sentence to solve it.
1,500
|------------|-----|
x 346
Hopefully those help the students with algebraic thinking.
Tags: math, elementary, algebra
Missing addend with x or a box?
Recently I've been writing worksheets for fourth grade... Later this year, I will make this collection available for whoever wishes to buy it. But for now, it's a work in progress.
One topic I was pondering a lot was whether to include problems such as
15 + x = 30 into the worksheets.
Or, 234 + x = 700
or x + 1,923 = 5,000.
I hope you get the idea; in elementary books you often see these kind of missing addend problems with a little box:
15 + = 30 or
= 30 or
234 + = 700 or
= 700 or
 + 1,923 = 5,000.
+ 1,923 = 5,000.
Well, I decided for the x over the box! I don't think solving missing addend problems with subtraction is too difficult to learn on fourth grade; after all, students have been working with addition and subtraction connection from 1st grade on, right?
In my own old schoolbooks I actually see x from 3rd grade on.
I made several problems with charts such as
Hopefully those help the students with algebraic thinking.
Tags: math, elementary, algebra
One topic I was pondering a lot was whether to include problems such as
15 + x = 30 into the worksheets.
Or, 234 + x = 700
or x + 1,923 = 5,000.
I hope you get the idea; in elementary books you often see these kind of missing addend problems with a little box:
15 +
 = 30 or
= 30 or 234 +
 = 700 or
= 700 or + 1,923 = 5,000.
+ 1,923 = 5,000. Well, I decided for the x over the box! I don't think solving missing addend problems with subtraction is too difficult to learn on fourth grade; after all, students have been working with addition and subtraction connection from 1st grade on, right?
In my own old schoolbooks I actually see x from 3rd grade on.
I made several problems with charts such as
Write a missing addend sentence
and a subtraction sentence to solve it.
1,500
|------------|-----|
x 346
Hopefully those help the students with algebraic thinking.
Tags: math, elementary, algebra
Saturday, May 13, 2006
Happy Mother's Day
Mother's day is a time of commemoration and celebration for all the moms' in the world, so here is a special pop-up card that you can make for that very special woman in your life. This pop-up card is from Canon and comes with either a rose or gerbera bouquet with beautiful ribbons on the bottom and enough space for some heartfelt message, send this along with your present and mom will love it.




Pop-up Card Pattern - Download
Thursday, May 11, 2006
Examples of calculus use in medicine?
I got a question,
I suspect doctors don't actually use any calculus in their daily work with people. BUT, it is used in medical research and analysis.
For example, calculus concepts are applied in studying how medicines act in the body. I found an article called Half-life and Steady State that talks about how the patient might be taking a medication and all the same time the body is clearing the previous doses... Eventually there comes a "steady state" where the amount of "the amount of drug going in is the same as the amount of drug getting taken out."
Or, from www.math.gatech.edu/~bourbaki/MapleProjects.html
we find that calculus is used to find out the rate of change of the surface area for a rapidly growing adolescent. This ties in with medicine in the fact that sometimes the drug dosage depends on the size of the individual, and the surface area is one way to measure the size of a person.
BIOLOGY and MEDICINE research abound with mathematical models, and calculus is an essential tool for analyzing those models. I personally have not studied these in-depth, but taking a peek in a Calculus for Biology and Medicine course syllabus from a certain community college we find that on that course the students;
Calculus is simply an indispesable tool for modern science.
Tags: math, calculus
"I am supposed to teach my calculus class one lesson.
That lesson has to be on something that can be applied
to whatever I am hoping to major in. I am planning on
studying pre-med to become a doctor. Could you tell
me how doctors apply math learned in calculus 1?"
I suspect doctors don't actually use any calculus in their daily work with people. BUT, it is used in medical research and analysis.
For example, calculus concepts are applied in studying how medicines act in the body. I found an article called Half-life and Steady State that talks about how the patient might be taking a medication and all the same time the body is clearing the previous doses... Eventually there comes a "steady state" where the amount of "the amount of drug going in is the same as the amount of drug getting taken out."
QUOTE
Many drug effects occur primarily when the blood level of the drug is either going up or going down. When the drug reaches steady state, these effects can be either attenuated or completely absent. For those of you who are familiar with calculus, one way to understand this is that these effects only take place if there is a first derivative other than zero.
Or, from www.math.gatech.edu/~bourbaki/MapleProjects.html
we find that calculus is used to find out the rate of change of the surface area for a rapidly growing adolescent. This ties in with medicine in the fact that sometimes the drug dosage depends on the size of the individual, and the surface area is one way to measure the size of a person.
QUOTE
"This worksheet, provides a correlation between height, weight, and surface area
for humans as determined by the commonly used West Nomogram. Additionally, partial derivatives (and the chain-rule) are used to find the rate of change of the
surface area for a rapidly growing adolescent."
BIOLOGY and MEDICINE research abound with mathematical models, and calculus is an essential tool for analyzing those models. I personally have not studied these in-depth, but taking a peek in a Calculus for Biology and Medicine course syllabus from a certain community college we find that on that course the students;
QUOTE
1. Analyze allometric models.
2. Analyze models in cell diffusion.
3. Analyze models in population growth models.
4. Analyze models in population biology for interacting species.
5. Analyze models for respiration and control of respiration.
6. Analyze models for cardiac dynamics and control of heart rhythms.
7. Analyze models for neuron dynamics.
8. Analyze models in pharmacology.
9. Utilize a computer-algebra system in a model's analysis.
Calculus is simply an indispesable tool for modern science.
Tags: math, calculus
Examples of calculus use in medicine?
I got a question,
I suspect doctors don't actually use any calculus in their daily work with people. BUT, it is used in medical research and analysis.
For example, calculus concepts are applied in studying how medicines act in the body. I found an article called Half-life and Steady State that talks about how the patient might be taking a medication and all the same time the body is clearing the previous doses... Eventually there comes a "steady state" where the amount of "the amount of drug going in is the same as the amount of drug getting taken out."
Or, from www.math.gatech.edu/~bourbaki/MapleProjects.html
we find that calculus is used to find out the rate of change of the surface area for a rapidly growing adolescent. This ties in with medicine in the fact that sometimes the drug dosage depends on the size of the individual, and the surface area is one way to measure the size of a person.
BIOLOGY and MEDICINE research abound with mathematical models, and calculus is an essential tool for analyzing those models. I personally have not studied these in-depth, but taking a peek in a Calculus for Biology and Medicine course syllabus from a certain community college we find that on that course the students;
Calculus is simply an indispesable tool for modern science.
Tags: math, calculus
"I am supposed to teach my calculus class one lesson.
That lesson has to be on something that can be applied
to whatever I am hoping to major in. I am planning on
studying pre-med to become a doctor. Could you tell
me how doctors apply math learned in calculus 1?"
I suspect doctors don't actually use any calculus in their daily work with people. BUT, it is used in medical research and analysis.
For example, calculus concepts are applied in studying how medicines act in the body. I found an article called Half-life and Steady State that talks about how the patient might be taking a medication and all the same time the body is clearing the previous doses... Eventually there comes a "steady state" where the amount of "the amount of drug going in is the same as the amount of drug getting taken out."
QUOTE
Many drug effects occur primarily when the blood level of the drug is either going up or going down. When the drug reaches steady state, these effects can be either attenuated or completely absent. For those of you who are familiar with calculus, one way to understand this is that these effects only take place if there is a first derivative other than zero.
Or, from www.math.gatech.edu/~bourbaki/MapleProjects.html
we find that calculus is used to find out the rate of change of the surface area for a rapidly growing adolescent. This ties in with medicine in the fact that sometimes the drug dosage depends on the size of the individual, and the surface area is one way to measure the size of a person.
QUOTE
"This worksheet, provides a correlation between height, weight, and surface area
for humans as determined by the commonly used West Nomogram. Additionally, partial derivatives (and the chain-rule) are used to find the rate of change of the
surface area for a rapidly growing adolescent."
BIOLOGY and MEDICINE research abound with mathematical models, and calculus is an essential tool for analyzing those models. I personally have not studied these in-depth, but taking a peek in a Calculus for Biology and Medicine course syllabus from a certain community college we find that on that course the students;
QUOTE
1. Analyze allometric models.
2. Analyze models in cell diffusion.
3. Analyze models in population growth models.
4. Analyze models in population biology for interacting species.
5. Analyze models for respiration and control of respiration.
6. Analyze models for cardiac dynamics and control of heart rhythms.
7. Analyze models for neuron dynamics.
8. Analyze models in pharmacology.
9. Utilize a computer-algebra system in a model's analysis.
Calculus is simply an indispesable tool for modern science.
Tags: math, calculus
Wednesday, May 10, 2006
Sonic the Hedgehog Papercraft
E3 is underway and Sony has finally announced a worldwide launch date (Nov. 17) and pricing ($499 & $599) for its upcoming Playstation 3 video game console. As with the release of the PS3, Sega is also in the works for a brand new Sonic title that will take advantage of these next gen systems, so while we are waiting for it, here is a Sonic the Hedgehog papercraft from the Sega Sonicteam that you might wanna try. This paper model is no longer available for download at the Sonicteam web site so I've uploaded it to a free file server for your convenience. The files are in JPG format with three parts for the pattern, one for the instructions, and are zipped. Enjoy!






Sonic the Hedgehog [via MediaFire]
Shadow the Hedgehog [Related Post]






Sonic the Hedgehog [via MediaFire]
Shadow the Hedgehog [Related Post]
I hate math but don't want to anymore...
I want to blog again on Julie's great Living Math website. You can really get help there if you're one of those who don't care for math, or even hate math, but want to get rid of that feeling.
She has suggestions on how to start teaching "living math" - teaching math in a way that makes connections to real life, takes away the dryness of it, takes away the 'kill' from "drill and kill" (note that drill in itself doesn't have to be always bad - drill is a tool amongst many), etc.
On this page, Julie has book suggestions to many different situations... Consider finding one or a few (from library or bookstore). Reading a math book that's not a school book can do SO much good! For your kids too!
As you probably know, I wish everyone would get to know some of the interesting, fascinating, fun, curious aspects of mathematics. Or, get to know a bit of math history. You don't get those in school books. Or, finally learn why things work.
These things CAN change your attitude towards math, and then you can relay it to your children.
Julie has already done the work of listing these books; I'm glad for that. Does any of the following apply to you? She says:
Now just go jot down a few of those books, run to library (or bookstore), and get started:
Living Math: Starting out suggestions
Tags: math, mathematics, teaching
She has suggestions on how to start teaching "living math" - teaching math in a way that makes connections to real life, takes away the dryness of it, takes away the 'kill' from "drill and kill" (note that drill in itself doesn't have to be always bad - drill is a tool amongst many), etc.
On this page, Julie has book suggestions to many different situations... Consider finding one or a few (from library or bookstore). Reading a math book that's not a school book can do SO much good! For your kids too!
As you probably know, I wish everyone would get to know some of the interesting, fascinating, fun, curious aspects of mathematics. Or, get to know a bit of math history. You don't get those in school books. Or, finally learn why things work.
These things CAN change your attitude towards math, and then you can relay it to your children.
Julie has already done the work of listing these books; I'm glad for that. Does any of the following apply to you? She says:
QUOTE
Are you a parent wanting to increase your understanding of *why* math is important?
Do you need understanding to increase your own enthusiasm, creativity and motivation?
Are you a parent wanting to understand why mathematics can seem difficult, wanting to gain reassurance that the "math gene" didn't skip your family?
Are you wanting to be convinced that mathematics can be a readable, living, subject?
Are you wanting to introduce mathematics to your children and adolescents in a vibrant, visual way, expanding the understanding of what mathematics is beyond arithmetic
~~ allowing them to *hear* the "music" they might play some day?
Are you a parent with a child that is "burned out" and needs a math reading break?
Now just go jot down a few of those books, run to library (or bookstore), and get started:
Living Math: Starting out suggestions
Tags: math, mathematics, teaching
I hate math but don't want to anymore...
I want to blog again on Julie's great Living Math website. You can really get help there if you're one of those who don't care for math, or even hate math, but want to get rid of that feeling.
She has suggestions on how to start teaching "living math" - teaching math in a way that makes connections to real life, takes away the dryness of it, takes away the 'kill' from "drill and kill" (note that drill in itself doesn't have to be always bad - drill is a tool amongst many), etc.
On this page, Julie has book suggestions to many different situations... Consider finding one or a few (from library or bookstore). Reading a math book that's not a school book can do SO much good! For your kids too!
As you probably know, I wish everyone would get to know some of the interesting, fascinating, fun, curious aspects of mathematics. Or, get to know a bit of math history. You don't get those in school books. Or, finally learn why things work.
These things CAN change your attitude towards math, and then you can relay it to your children.
Julie has already done the work of listing these books; I'm glad for that. Does any of the following apply to you? She says:
Now just go jot down a few of those books, run to library (or bookstore), and get started:
Living Math: Starting out suggestions
Tags: math, mathematics, teaching
She has suggestions on how to start teaching "living math" - teaching math in a way that makes connections to real life, takes away the dryness of it, takes away the 'kill' from "drill and kill" (note that drill in itself doesn't have to be always bad - drill is a tool amongst many), etc.
On this page, Julie has book suggestions to many different situations... Consider finding one or a few (from library or bookstore). Reading a math book that's not a school book can do SO much good! For your kids too!
As you probably know, I wish everyone would get to know some of the interesting, fascinating, fun, curious aspects of mathematics. Or, get to know a bit of math history. You don't get those in school books. Or, finally learn why things work.
These things CAN change your attitude towards math, and then you can relay it to your children.
Julie has already done the work of listing these books; I'm glad for that. Does any of the following apply to you? She says:
QUOTE
Are you a parent wanting to increase your understanding of *why* math is important?
Do you need understanding to increase your own enthusiasm, creativity and motivation?
Are you a parent wanting to understand why mathematics can seem difficult, wanting to gain reassurance that the "math gene" didn't skip your family?
Are you wanting to be convinced that mathematics can be a readable, living, subject?
Are you wanting to introduce mathematics to your children and adolescents in a vibrant, visual way, expanding the understanding of what mathematics is beyond arithmetic
~~ allowing them to *hear* the "music" they might play some day?
Are you a parent with a child that is "burned out" and needs a math reading break?
Now just go jot down a few of those books, run to library (or bookstore), and get started:
Living Math: Starting out suggestions
Tags: math, mathematics, teaching
Monday, May 8, 2006
Motivation to study math
I felt very inspired by the story I posted last time, about the 12 kids who wanted to have a class to study arithmetic, and then finished 6 years worth of school math in 20 weeks - meaning they had 20 contact hours with the teacher, and who knows how many hours spent on homework.
They studied the four basic operations, fractions, decimals, percent, and square root. I figure they didn't go into algebra - just arithmetic.
Like it said in the article, the material itself is not incredibly difficult, once your mind has developed to handle these concepts.
The story shows how much motivation (and subsequent hard work) can do. So how could we increase our students' motivation to study math?
I feel it is important to PREVENT the student's feeling of, "I hate math" or "I don't like math" that the traditional math instruction seems to produce. Little kids usually like learning about different things. Somehow we must keep that enthusiasm going strong.
For example:
I guess this was quite a rant; I'm sorry if it was just repeating 'old truths'. Hopefully it's helpful to someone out there!
Tags: math, mathematics, philosophy, teaching
They studied the four basic operations, fractions, decimals, percent, and square root. I figure they didn't go into algebra - just arithmetic.
Like it said in the article, the material itself is not incredibly difficult, once your mind has developed to handle these concepts.
The story shows how much motivation (and subsequent hard work) can do. So how could we increase our students' motivation to study math?
I feel it is important to PREVENT the student's feeling of, "I hate math" or "I don't like math" that the traditional math instruction seems to produce. Little kids usually like learning about different things. Somehow we must keep that enthusiasm going strong.
For example:
- As much as possible, the teacher should love and like math. Your motivation and enthusiasm spreads to your students.
If you need to, first learn math yourself - including WHY things work. Find something you like about math. Read books perhaps - and I don't mean school books but 'fun' math books, books about beauty of math, or math history, or something different - REAL. Julie at LivingMath.net has an extensive book list. Find something for the kids, too. For example, King Joe books inspire 2-4th graders and incorporate math terminology into interesting stories (but are pricey). - I've said this before; I guess I say it again: show your students how math is part of your daily life. You're cooking; you're estimating; you're figuring time intervals; you're shopping; planning a garden; doing taxes; you're figuring how many of something you need etc. Share all that.
- Never become a slave to your math curriculum. It is a TOOL for learning - not the goal. The goal is to learn.
- Set up definite goals for math learning. Heather Madrone calls it her "Non-Curriculum". For example, you might have goals on your list such as:
1) learning concept of number, concept of addition and subtraction using numbers 0-100.
2) basic place value within 0-100.
3) start memorizing basic facts.
4) Explore concepts of fraction, multiplication, division, measuring.
...and then you can use math books, games, manipulatives, the curriculum you have, workbooks, worksheets etc. to achieve the goals.
I guess this was quite a rant; I'm sorry if it was just repeating 'old truths'. Hopefully it's helpful to someone out there!
Tags: math, mathematics, philosophy, teaching
Motivation to study math
I felt very inspired by the story I posted last time, about the 12 kids who wanted to have a class to study arithmetic, and then finished 6 years worth of school math in 20 weeks - meaning they had 20 contact hours with the teacher, and who knows how many hours spent on homework.
They studied the four basic operations, fractions, decimals, percent, and square root. I figure they didn't go into algebra - just arithmetic.
Like it said in the article, the material itself is not incredibly difficult, once your mind has developed to handle these concepts.
The story shows how much motivation (and subsequent hard work) can do. So how could we increase our students' motivation to study math?
I feel it is important to PREVENT the student's feeling of, "I hate math" or "I don't like math" that the traditional math instruction seems to produce. Little kids usually like learning about different things. Somehow we must keep that enthusiasm going strong.
For example:
I guess this was quite a rant; I'm sorry if it was just repeating 'old truths'. Hopefully it's helpful to someone out there!
Tags: math, mathematics, philosophy, teaching
They studied the four basic operations, fractions, decimals, percent, and square root. I figure they didn't go into algebra - just arithmetic.
Like it said in the article, the material itself is not incredibly difficult, once your mind has developed to handle these concepts.
The story shows how much motivation (and subsequent hard work) can do. So how could we increase our students' motivation to study math?
I feel it is important to PREVENT the student's feeling of, "I hate math" or "I don't like math" that the traditional math instruction seems to produce. Little kids usually like learning about different things. Somehow we must keep that enthusiasm going strong.
For example:
- As much as possible, the teacher should love and like math. Your motivation and enthusiasm spreads to your students.
If you need to, first learn math yourself - including WHY things work. Find something you like about math. Read books perhaps - and I don't mean school books but 'fun' math books, books about beauty of math, or math history, or something different - REAL. Julie at LivingMath.net has an extensive book list. Find something for the kids, too. For example, King Joe books inspire 2-4th graders and incorporate math terminology into interesting stories (but are pricey). - I've said this before; I guess I say it again: show your students how math is part of your daily life. You're cooking; you're estimating; you're figuring time intervals; you're shopping; planning a garden; doing taxes; you're figuring how many of something you need etc. Share all that.
- Never become a slave to your math curriculum. It is a TOOL for learning - not the goal. The goal is to learn.
- Set up definite goals for math learning. Heather Madrone calls it her "Non-Curriculum". For example, you might have goals on your list such as:
1) learning concept of number, concept of addition and subtraction using numbers 0-100.
2) basic place value within 0-100.
3) start memorizing basic facts.
4) Explore concepts of fraction, multiplication, division, measuring.
...and then you can use math books, games, manipulatives, the curriculum you have, workbooks, worksheets etc. to achieve the goals.
I guess this was quite a rant; I'm sorry if it was just repeating 'old truths'. Hopefully it's helpful to someone out there!
Tags: math, mathematics, philosophy, teaching
Saturday, May 6, 2006
How far you can go when you're REALLY motivated
Just found this... decided that it's good reading for all. When you have lots of motivation, like these kids did, you can learn and will learn - math or anything else. It may not be easy at all times, but the motivation will make you persistent so you will eventually learn.
A certain arithmetic class at Sudbury Valley School
A certain arithmetic class at Sudbury Valley School
QUOTE
"Because everyone knows," he answered, "that the subject matter itself isn't that hard. What's hard, virtually impossible, is beating it into the heads of youngsters who hate every step. The only way we have a ghost of a chance is to hammer away at the stuff bit by bit every day for years. Even then it does not work. Most of the sixth graders are mathematical illiterates. Give me a kid who wants to learn the stuff -- well, twenty hours or so makes sense."
How far you can go when you're REALLY motivated
Just found this... decided that it's good reading for all. When you have lots of motivation, like these kids did, you can learn and will learn - math or anything else. It may not be easy at all times, but the motivation will make you persistent so you will eventually learn.
A certain arithmetic class at Sudbury Valley School
A certain arithmetic class at Sudbury Valley School
QUOTE
"Because everyone knows," he answered, "that the subject matter itself isn't that hard. What's hard, virtually impossible, is beating it into the heads of youngsters who hate every step. The only way we have a ghost of a chance is to hammer away at the stuff bit by bit every day for years. Even then it does not work. Most of the sixth graders are mathematical illiterates. Give me a kid who wants to learn the stuff -- well, twenty hours or so makes sense."
Friday, May 5, 2006
The daily grind of math - making connections
I wanted to briefly touch some more on this topic of "grind" or daily grind that learning math might sometimes become that I mentioned briefly last time.
I didn't mean to imply that learning to add, subtract, multiply, and divide whole numbers, decimals, fractions, percents, numbers with exponents, and integers has to be such a boring task. You can avoid that type of feeling.
One way:
Make connections between the concepts. Don't make math appear as fairly separate compartments of "fractions" and "decimals" and "percents" and "geometry".
I sometimes wonder how students feel when every year (on 4th, 5th, 6th, 7th, and 8th grade) they have a chapter on fractions, a chapter on decimals, a chapter on whole numbers, a chapter on geometry... Almost the same thing over and over.
We need to make sure they can see how these things connect.
An example:
A jogging track is 3.5 km long. If you jog 2/5 of it, how far was that? How many percent of the track is that?
Can your student solve it? What do you think, when are they ready for this sort of problem?
Tags: math, mathematics
I didn't mean to imply that learning to add, subtract, multiply, and divide whole numbers, decimals, fractions, percents, numbers with exponents, and integers has to be such a boring task. You can avoid that type of feeling.
One way:
Make connections between the concepts. Don't make math appear as fairly separate compartments of "fractions" and "decimals" and "percents" and "geometry".
I sometimes wonder how students feel when every year (on 4th, 5th, 6th, 7th, and 8th grade) they have a chapter on fractions, a chapter on decimals, a chapter on whole numbers, a chapter on geometry... Almost the same thing over and over.
We need to make sure they can see how these things connect.
An example:
A jogging track is 3.5 km long. If you jog 2/5 of it, how far was that? How many percent of the track is that?
|--------------------------------------------|
0 3.5 km
|--------|--------|--------|--------|--------|
0 1
|--------------------------------------------|
0 100%
Can your student solve it? What do you think, when are they ready for this sort of problem?
Tags: math, mathematics
The daily grind of math - making connections
I wanted to briefly touch some more on this topic of "grind" or daily grind that learning math might sometimes become that I mentioned briefly last time.
I didn't mean to imply that learning to add, subtract, multiply, and divide whole numbers, decimals, fractions, percents, numbers with exponents, and integers has to be such a boring task. You can avoid that type of feeling.
One way:
Make connections between the concepts. Don't make math appear as fairly separate compartments of "fractions" and "decimals" and "percents" and "geometry".
I sometimes wonder how students feel when every year (on 4th, 5th, 6th, 7th, and 8th grade) they have a chapter on fractions, a chapter on decimals, a chapter on whole numbers, a chapter on geometry... Almost the same thing over and over.
We need to make sure they can see how these things connect.
An example:
A jogging track is 3.5 km long. If you jog 2/5 of it, how far was that? How many percent of the track is that?
Can your student solve it? What do you think, when are they ready for this sort of problem?
Tags: math, mathematics
I didn't mean to imply that learning to add, subtract, multiply, and divide whole numbers, decimals, fractions, percents, numbers with exponents, and integers has to be such a boring task. You can avoid that type of feeling.
One way:
Make connections between the concepts. Don't make math appear as fairly separate compartments of "fractions" and "decimals" and "percents" and "geometry".
I sometimes wonder how students feel when every year (on 4th, 5th, 6th, 7th, and 8th grade) they have a chapter on fractions, a chapter on decimals, a chapter on whole numbers, a chapter on geometry... Almost the same thing over and over.
We need to make sure they can see how these things connect.
An example:
A jogging track is 3.5 km long. If you jog 2/5 of it, how far was that? How many percent of the track is that?
|--------------------------------------------|
0 3.5 km
|--------|--------|--------|--------|--------|
0 1
|--------------------------------------------|
0 100%
Can your student solve it? What do you think, when are they ready for this sort of problem?
Tags: math, mathematics
Wednesday, May 3, 2006
Legend of Zelda Papercraft - Four Swords Link
For video gamers E3 is on the of the most anticipated yearly gaming convention comprised of the computer and video game industry. This is the time of year when video game developers show off their games and other game-related hardware. E3 2006 is just a few days away (May 10 -12, Los Angeles Convention Center), and to get things rolling here is the Legend of Zelda papercraft - Four Swords Link.
This paper model is the official Nintendo release back in 2004 via the "Famitsu Cube and Advance" magazine. It's out of print now and only a handful can be found on the net (eBay?). It was created by Chokipeta and commisioned by Nintendo, as you might remember Chokipeta is also the one that created the Shigaraki racoon dog paper model. The files are in PDF format, two for the pattern, one for the instructions and zipped. So without further ado...






Legend of Zelda Papercraft - Four Swords Link [via Mediafire]
This paper model is the official Nintendo release back in 2004 via the "Famitsu Cube and Advance" magazine. It's out of print now and only a handful can be found on the net (eBay?). It was created by Chokipeta and commisioned by Nintendo, as you might remember Chokipeta is also the one that created the Shigaraki racoon dog paper model. The files are in PDF format, two for the pattern, one for the instructions and zipped. So without further ado...






Legend of Zelda Papercraft - Four Swords Link [via Mediafire]
Labels:
chokipeta,
Nintendo,
Paper Models,
Papercraft,
videogame
Subscribe to:
Comments (Atom)
